 Time-dependent CP violation & Unitarity Triangle angles
Time-dependent CP violation & Unitarity Triangle angles
 Time-dependent CP violation & Unitarity Triangle angles
Time-dependent CP violation & Unitarity Triangle angles
|
Legend: if not stated otherwise,
We use Combos v3.20 (homepage, manual) for the rescaling of the experimental results to common sets of input parameters.
The experimental results have been rescaled to a common set of input parameters (see table below).
| Parameter | Value | Reference |
|---|---|---|
| τ(Bd) | (1.519 ± 0.007) ps | HFAG - Oscillations/Lifetime |
| Δmd | (0.507 ± 0.004) ps−1 | HFAG - Oscillations/Lifetime |
| ΔΓd/Γd | 0.015 ± 0.018 | HFAG - Oscillations/Lifetime |
|
|A⊥|2 (CP-odd fraction in B0→ J/ψK* CP sample) |
0.233 ± 0.010 ± 0.005 |
BaBar: PRD 76 (2007) 031102
N(BB)=232m |
| 0.195 ± 0.012 ± 0.008 |
Belle: PRL 95 (2005) 091601
N(BB)=275m |
|
| 0.215 ± 0.032 ± 0.006 |
CDF: PRL 94 (2005) 101803 (*)
∫Ldt=0.3 fb−1 |
|
| 0.183 ± 0.013 ± 0.025 |
D0: PRL 102 (2009) 032001
∫Ldt=2.8 fb−1 |
|
| 0.178 ± 0.022 ± 0.017 |
LHCb: LHCb-CONF-2011-002
∫Ldt=0.4 fb−1 |
|
| 0.213 ± 0.008 |
Average
χ2 = 7.5/4 dof (CL=0.11 ⇒ 1.6σ) |
(*) We do not include an unpublished CDF preliminary result from 2007.
Additional note on commonly treated (correlated) systematic effects:
We obtain for sin(2β) ≡ sin(2φ1) in the different decay modes:
| Parameter: sin(2β) ≡ sin(2φ1) | ||||
|---|---|---|---|---|
| Mode | BaBar | Belle | Average | Reference |
| Charmonium: | N(BB)=465M | N(BB)=772M | ||
| J/ψKS (ηCP=-1) | 0.657 ± 0.036 ± 0.012 | 0.670 ± 0.029 ± 0.013 | 0.665 ± 0.024 (0.023stat-only) |
BaBar (PRD 79 (2009) 072009)
Belle (PRL 108 (2012) 171802) |
| J/ψKL (ηCP=+1) | 0.694 ± 0.061 ± 0.031 | 0.642 ± 0.047 ± 0.021 | 0.663 ± 0.041 (0.037stat-only) |
|
| J/ψK0 | 0.666 ± 0.031 ± 0.013 | - | 0.665 ± 0.022 (0.019stat-only) |
|
| ψ(2S)KS (ηCP=-1) | 0.897 ± 0.100 ± 0.036 | 0.738 ± 0.079 ± 0.036 | 0.807 ± 0.067 (0.062stat-only) |
|
| ψ(nS)K0 | - | - | 0.676 ± 0.021 (0.018stat-only) |
|
| χc1KS (ηCP=-1) | 0.614 ± 0.160 ± 0.040 | 0.640 ± 0.117 ± 0.040 | 0.632 ± 0.099 (0.094stat-only) |
|
| ηcKS (ηCP=-1) | 0.925 ± 0.160 ± 0.057 | - | - | BaBar (PRD 79 (2009) 072009) |
| J/ψK*0 (K*0 → KSπ0) (ηCP= 1-2|A⊥|2) | 0.601 ± 0.239 ± 0.087 | - | ||
| All charmonium | 0.687 ± 0.028 ± 0.012 | 0.667 ± 0.023 ± 0.012 | 0.677 ± 0.020 (0.018stat-only) |
CL = 0.57 |
| χc0KS (ηCP=+1) |
0.69 ± 0.52 ± 0.04 ± 0.07 (*)
N(BB)=383M |
- | - | BaBar (PRD 80 (2009) 112001) |
| J/ψKS, J/ψ → hadrons (ηCP=+1) |
1.56 ± 0.42 ± 0.21 (**)
N(BB)=88M |
- | - | BaBar (PRD 69 (2004) 052001) |
| All charmonium (incl. χc0KS etc.) |
0.691 ± 0.031
(0.028stat-only) |
0.667 ± 0.023 ± 0.012 | 0.679 ± 0.020 (0.018stat-only) |
CL = 0.28 |
(*) The BABAR result on χc0KS comes from the time-dependent Dalitz plot analysis of B0 → π+π−KS. The third uncertainty is due to the Dalitz model.
(**) BaBar (PRD 69 (2004) 052001) uses "hadronic and previously unused muonic decays of the J/ψ". We neglect a small possible correlation of this result with the main BaBar result that could be caused by reprocessing of the data.
Including earlier sin(2β) ≡ sin(2φ1) measurements, as well as a recent result from LHCb, using Bd → J/ψKS decays, and a measurement by Belle using Υ(5S) data and B-π tagging:
| Parameter: sin(2β) ≡ sin(2φ1) | ||||
|---|---|---|---|---|
| Experiment | Value | Reference | ||
| ALEPH | 0.84 +0.82−1.04 ± 0.16 | PL B492 (2000) 259-274 | ||
| OPAL | 3.2 +1.8−2.0 ± 0.5 | EPJ C5 (1998) 379-388 | ||
| CDF (full Run I) | 0.79 +0.41−0.44(stat+syst) | PRD 61 (2000) 072005 | ||
| LHCb (1.0/fb) | 0.73 ± 0.07 ± 0.04 | LHCb-PAPER-2012-035 | ||
| Belle (121/fb Υ(5S) data) | 0.57 ± 0.58 ± 0.06 | PRL 108 (2012) 171801 | ||
we find the only slightly modified average:
| Parameter: sin(2β) ≡ sin(2φ1) | ||||
|---|---|---|---|---|
| All charmonium | 0.682 ± 0.019 (0.017stat-only) | CL = 0.25 | ||
from which we obtain the following solutions for β ≡ φ1 (in [0, π])
| β ≡ φ1 = (21.5 +0.8−0.7)° | or | β ≡ φ1 = (68.5 +0.7−0.8)° |
Plots:
| Average of sin(2β) ≡ sin(2φ1) from all experiments. |
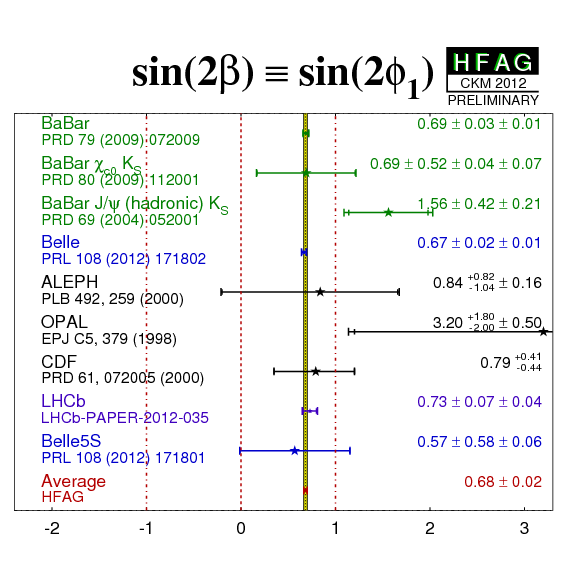
eps.gz png |
|||
| Averages of sin(2β) ≡ sin(2φ1) and C=-A from the B factories. |
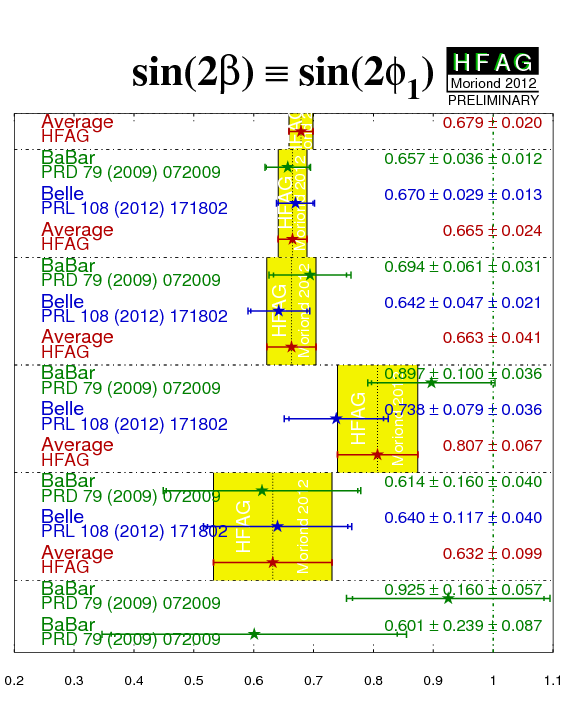
eps.gz png |
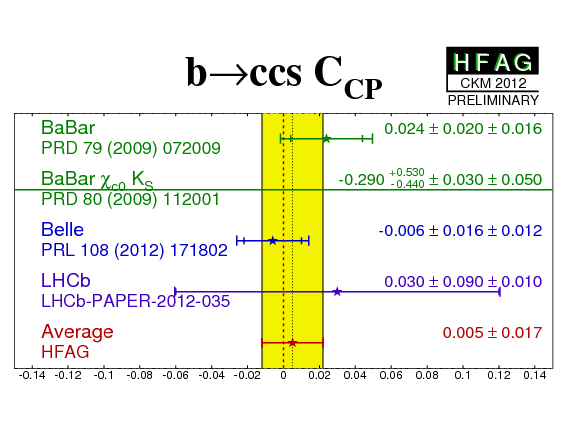
eps.gz png |
||
| Constraint on the ρ-bar-η-bar plane: |
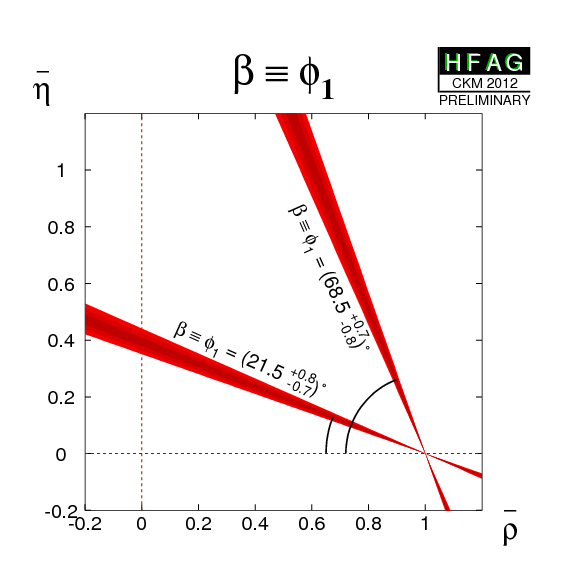
eps.gz png |
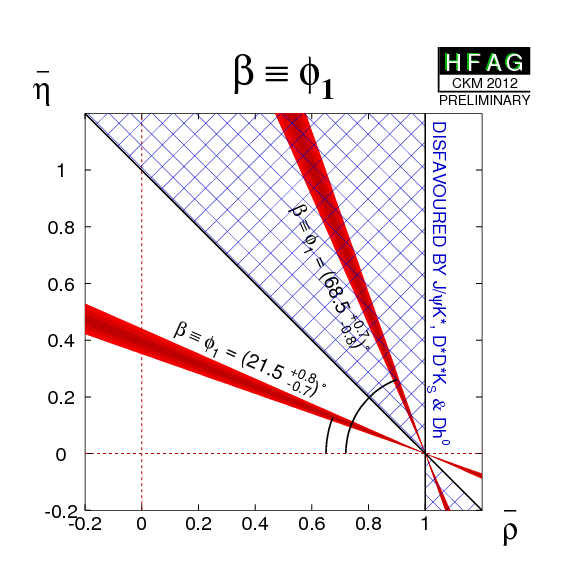
eps.gz png |
||
|
Constraining the Unitarity Triangle (ρ, η):
Visit the CKMfitter and UTfit sites for results on global CKM fits using different fit techniques and input quantities. |
Historically the experiments determined |λ| for the charmonium modes; more recently the parameters C = −A = (1−|λ|2)/(1+|λ|2) are being used, as they are in all other time-dependent CP analyses. We recompute C from |λ| (from the BaBar results) for the following averages.
| Parameter: C=−A (if not stated otherwise) | ||||
|---|---|---|---|---|
| Mode | BaBar | Belle | Average | Reference |
| Charmonium: | N(BB)=465M | N(BB)=772M | ||
| J/ψKS | 0.026 ± 0.025 ± 0.016 | 0.015 ± 0.021 +0.023−0.045 | 0.024 ± 0.026 (0.016stat-only) |
BaBar (PRD 79 (2009) 072009)
Belle (PRL 108 (2012) 171802) |
| J/ψKL | −0.033 ± 0.050 ± 0.027 | −0.019 ± 0.026 +0.041−0.017 | −0.023 ± 0.030 (0.023stat-only) |
|
| J/ψK0 | 0.016 ± 0.023 ± 0.018 | - | 0.006 ± 0.021 (0.013stat-only) |
|
| ψ(2S)KS | 0.089 ± 0.076 ± 0.020 | −0.104 ± 0.055 +0.027−0.047 | −0.009 ± 0.055 (0.045stat-only) |
|
| ψ(nS)K0 | - | - | 0.005 ± 0.020 (0.013stat-only) |
|
| χc1KS | 0.129 ± 0.109 ± 0.025 | 0.017 ± 0.083 +0.026−0.046 | 0.066 ± 0.074 (0.066stat-only) |
|
| ηcKS | 0.080 ± 0.124 ± 0.029 | - | - | BaBar (PRD 79 (2009) 072009) |
| J/ψK*0 (K*0 → KSπ0) | 0.025 ± 0.083 ± 0.054 | - | ||
| All charmonium | 0.024 ± 0.020 ± 0.016 | −0.006 ± 0.016 ± 0.012 | 0.006 ± 0.017 (0.012stat-only) |
CL = 0.29 |
| χc0KS (ηCP=+1) | −0.29 +0.53−0.44 ± 0.03 ± 0.05 (*) | - | - | BaBar (PRD 80 (2009) 112001) |
| All charmonium (incl. χc0KS) | 0.023 ± 0.025 (0.020stat-only) |
−0.006 ± 0.016 ± 0.012 | 0.005 ± 0.017 (0.012stat-only) |
CL = 0.47 |
(*) The BABAR result on χc0KS comes from the time-dependent Dalitz plot analysis of B0 → π+π−KS. The third uncertainty is due to the Dalitz model.
Including a recent result from LHCb, using Bd → J/ψKS decays:
| Parameter: sin(2β) ≡ sin(2φ1) | ||||
|---|---|---|---|---|
| Experiment | Value | Reference | ||
| LHCb (1.0/fb) | 0.03 ± 0.09 ± 0.01 | LHCb-PAPER-2012-035 | ||
The statistical correlation between the LHCb results for S and C is 0.42.
we find an average that is unchanged within the rounding:
| Parameter: C(b → c c-bar s) | ||||
|---|---|---|---|---|
| All charmonium | 0.005 ± 0.017 | |||
The BaBar and Belle collaborations have performed measurements of sin(2β) & cos(2β) ≡ sin(2φ1) & cos(2φ1) in time-dependent transversity analyses of the pseudoscalar to vector-vector decay B0→ J/ψK*, where cos(2β) ≡ cos(2φ1) enters as a factor in the interference between CP-even and CP-odd amplitudes. In principle, this analysis comes along with an ambiguity on the sign of cos(2β) ≡ cos(2φ1) due to an incomplete determination of the strong phases occurring in the three transversity amplitudes. BaBar resolves this ambiguity by inserting the known variation of the rapidly moving P-wave phase relative to the slowly moving S-wave phase with the invariant mass of the Kπ system in the vicinity of the K*(892) resonance. The result is in agreement with the prediction obtained from s-quark helicity conservation. It corresponds to Solution II defined by Suzuki, which is the phase convention used for the averages given here.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
| Experiment | sin(2β) ≡ sin(2φ1)J/ψK* | cos(2β) ≡ cos(2φ1)J/ψK* | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=88M |
−0.10 ± 0.57 ± 0.14 | 3.32 +0.76 −0.96 ± 0.27 | −0.37 (stat) | PRD 71, 032005 (2005) |
|
Belle
N(BB)=275M |
0.24 ± 0.31 ± 0.05 |
0.56 ± 0.79 ± 0.11
[using Solution II] |
0.22 (stat) | PRL 95 091601 (2005) |
| Average |
0.16 ± 0.28
χ2 = 0.3/1 dof (CL = 0.61 → 0.5σ) |
1.64 ± 0.62
χ2 = 4.7/1 dof (CL = 0.03 → 2.2σ) |
uncorrelated averages |
HFAG
See remark below table |
|
|
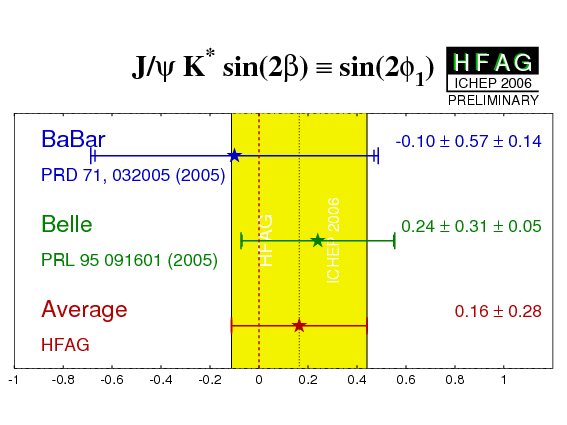
eps.gz png |
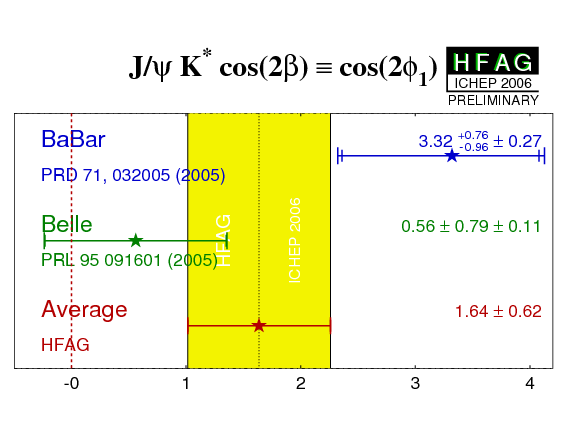
eps.gz png |
. | |
BaBar
find a confidence level for cos(2β)>0 of 89%.
Note that due to the strong non-Gaussian character of the BaBar measurement,
the interpretation of the average given above
has to be done with the greatest care.
We perform uncorrelated averages
(using the PDG prescription
for asymmetric errors).
The decays Bd → D(*)D(*)KS are dominated by the b → cc-bar s transition, and are therefore sensitive to 2β ≡ 2φ1. However, since the final state is not a CP eigenstate, extraction of the weak phases is difficult. Browder et al. have shown that terms sensitive to cos(2β) ≡ cos(2φ1) can be extracted from the analysis of Bd → D*D*KS decays (with some theoretical input).
Analysis of the Bd → D*D*KS decay has been performed by BaBar. and Belle.
The analyses proceed by dividing the Dalitz plot into two: m(D*+KS)2 > m(D*−KS)2 (ηy = +1) and m(D*+KS)2 < m(D*−KS)2 (ηy = -1). They then fit using a PDF where the time-dependent asymmetry (defined in the usual way as the difference between the time-dependent distributions of B0-tagged and B0-bar-tagged events, divided by their sum) is given by
| A(Δt) = ηy (Jc/J0) cos(ΔmdΔt) − [ (2Js1/J0)sin(2β) + ηy (2Js2/J0)cos(2β) ] sin(ΔmdΔt) |
The parameters J0, Jc, Js1 and Js2 are the integrals over the half-Dalitz plane m(D*+KS)2 < m(D*−KS)2 of the functions |a|2 + |a-bar|2, |a|2 - |a-bar|2, Re(a-bar a*) and Im(a-bar a*) respectively, where a and a-bar are the decay amplitudes of B0 → D*D*KS and B0-bar → D*D*KS respectively. The parameter Js2 (and hence Js2/J0) is predicted to be positive.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
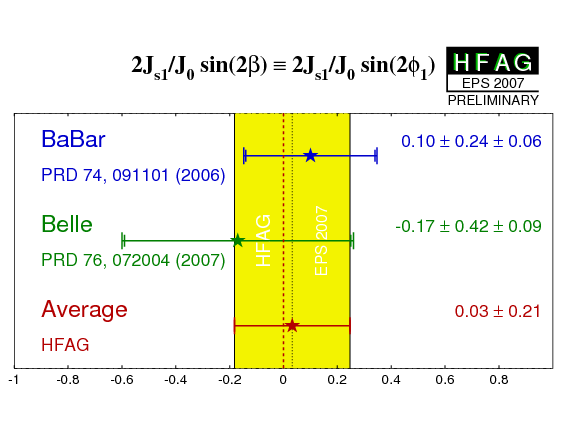
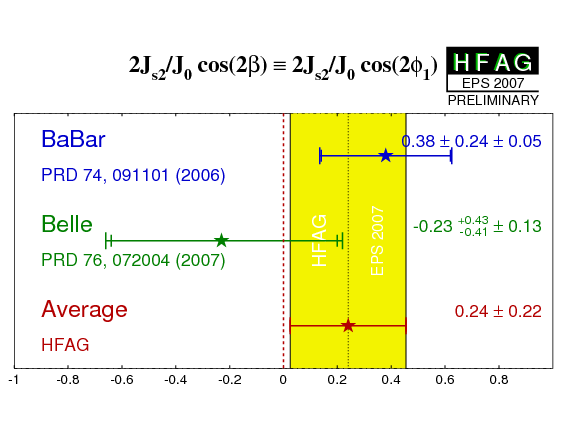
| Experiment | Jc/J0 | (2Js1/J0)sin(2β) ≡ (2Js1/J0)sin(2φ1) | (2Js2/J0)cos(2β) ≡ (2Js2/J0)cos(2φ1) | Correlation | Reference |
|---|---|---|---|---|---|
|
BaBar
N(BB)=230M |
0.76 ± 0.18 ± 0.07 | 0.10 ± 0.24 ± 0.06 | 0.38 ± 0.24 ± 0.05 | - | PRD 74, 091101 (2006) |
|
Belle
N(BB)=449M |
0.60 +0.25 −0.28 ± 0.08 | −0.17 ± 0.42 ± 0.09 | −0.23 +0.43 −0.41 ± 0.13 | - | PRD 76, 072004 (2007) |
| Average |
0.71 ± 0.16
χ2 = 0.2 (CL=0.63 ⇒ 0.5σ) |
0.03 ± 0.21
χ2 = 0.3 (CL=0.59 ⇒ 0.5σ) |
0.24 ± 0.22
χ2 = 1.4 (CL=0.23 ⇒ 1.2σ) |
uncorrelated averages | HFAG |
|
|
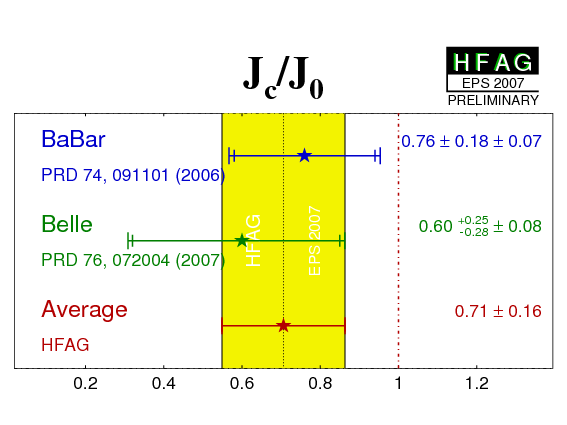
eps.gz png |
eps.gz png |
eps.gz png |
. | |
From the above result and the assumption that Js2>0, BaBar infer that cos(2β)>0 at the 94% confidence level.
Decays of the Bs meson via the b → cc-bar s transition probe φs, a CP violating phase related to Bs–Bs-bar mixing. An important difference with respect to the Bd–Bd-bar system, is that the value of ΔΓ is predicted to significantly non-zero, allowing information on φs to be extracted without tagging the flavour of the decaying B meson. Within the Standard Model, φs is predicted to be very small, O(λ2).
The vector-vector final state J/ψ φ contains mixtures of polarization amplitudes: the CP-odd A⊥, and the CP-even A0 and A||. These terms need to be disentangled, using the angular distributions, in order to extract φs, and their interference provides additional sensitivity. The sensitivity to φs depends strongly on ΔΓ, and less strongly on the perpendicularly polarized fraction, |A⊥|2.
|
In this discussion we make the approximation φs ≈ −2βs where φs ≡ arg[ − M12 / Γ12 ] and 2βs ≡ 2 arg[ − VtsVtb* / VcsVcb* ]. This is a reasonable approximation since, although the equality does not hold in the Standard Model, both are much smaller than the current experimental resolution, whereas new physics contributions add a phase φNP to φs and subtract the same phase from 2βs, so that the approximation remains valid. |
Measurements of φs, based on flavour-tagged analyses of Bs → J/ψ φ decays, have been performed by CDF, D0 and LHCb. ATLAS have performed an untagged analysis of the same decay. LHCb have in addition performed measurements of φs from Bs → J/ψ π+π−.
Averaging of the above results is being carried out by the HFAG lifetimes and oscillation group.
Bd decays to final states such as Dπ0 are governed by the b → cu-bar d transitions. If one chooses a final state which is a CP eigenstate, eg. DCPπ0, the usual time-dependence formulae are recovered, with the sine coefficient sensitive to sin(2β) ≡ sin(2φ1). Since there is no penguin contribution to these decays, there is even less associated theoretical uncertainty than for b → cc-bar s decays like Bd → J/ψ KS. See e.g. Fleischer, NPB 659, 321 (2003).
Results of such an analysis are available from BaBar. The decays Bd → Dπ0, Bd → Dη, Bd → Dω, Bd → D*π0 and Bd → D*η are used. The daughter decay D* → Dπ0 is used. The CP-even D decay to K+K− is used for all decay modes, with the CP-odd D decay to KSω also used in Bd → D(*)π0 and the additional CP-odd D decay to KSπ0 also used in Bd → Dω.
BaBar have performed separate fits for the cases where the intermediate D(*) decays to CP-even and CP-odd final states, since these receive different contributions fom subleading amplitudes in the Standard Model. Since the effects of these corrections are expected to be negligible (~0.02) compared to the current experimental uncertainty, they have also performed a fit with all decays combined.
| Mode | Experiment | −sin(2β) ≡ −sin(2φ1) | CCP | Correlation | Reference |
|---|---|---|---|---|---|
| D(*)CP+ h0 |
BaBar
N(BB)=383M |
−0.65 ± 0.26 ± 0.06 | −0.33 ± 0.19 ± 0.04 | 0.04 (stat) | PRL 99, 081801 (2007) |
| D(*)CP− h0 | −0.46 ± 0.46 ± 0.13 | −0.03 ± 0.28 ± 0.07 | −0.14 (stat) | ||
| D(*) h0 | −0.56 ± 0.23 ± 0.05 | −0.23 ± 0.16 ± 0.04 | −0.02 (stat) |
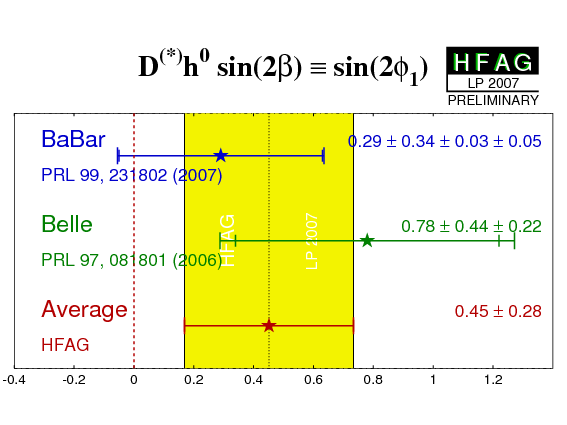
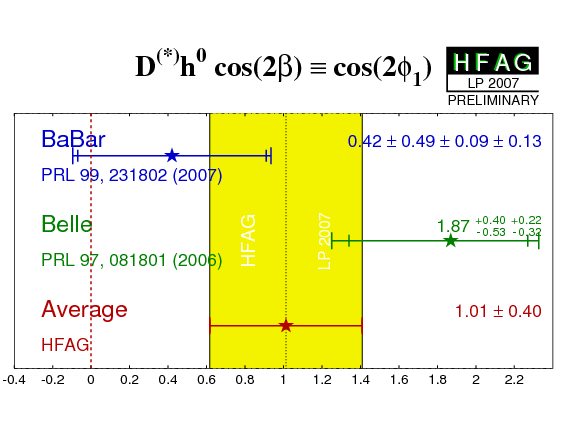
Bondar, Gershon and Krokovny have shown that when multibody D decays, such as D → KSπ+π− are used, a time-dependent analysis of the Dalitz plot of the D decay allows a direct determination of the weak phase: β ≡ φ1. Equivalently, both sin(2β) ≡ sin(2φ1) and cos(2β) ≡ cos(2φ1) can be measured. This information allows to resolve the ambiguity in the measurement of 2β ≡ 2φ1 from sin(2β) ≡ sin(2φ1) alone.
Results of such an analysis are available from both Belle and. BaBar. The decays Bd → Dπ0, Bd → Dη, Bd → Dω, Bd → D*π0 and Bd → D*η are used. The daughter decays are D* → Dπ0 and D → KSπ+π−. Note that BaBar quote uncertainties due to the D decay model separately from other systematic errors, while Belle do not.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
| Experiment | sin(2β) ≡ sin(2φ1) | cos(2β) ≡ cos(2φ1) | |λ| | Correlations | Reference |
|---|---|---|---|---|---|
|
BaBar
N(BB)=383M |
0.29 ± 0.34 ± 0.03 ± 0.05 | 0.42 ± 0.49 ± 0.09 ± 0.13 | 1.01 ± 0.08 ± 0.02 | (stat) | PRL 99, 231802 (2007) |
|
Belle
N(BB)=386M |
0.78 ± 0.44 ± 0.22 | 1.87 +0.40 −0.53 +0.22 −0.32 | - | - | PRL 97, 081801 (2006) |
| Average |
0.45 ± 0.28
χ2 = 0.7 (CL=0.41 ⇒ 0.8σ) |
1.01 ± 0.40
χ2 = 3.2 (CL=0.07 ⇒ 1.8σ) |
- | uncorrelated averages | HFAG |
|
|
eps.gz png |
eps.gz png |
. | ||
Interpretations:
Belle
determine the sign of cos(2φ)1 to be positive at 98.3% confidence level.
BaBar
favour the solution of β with cos(2β)>0 at 86% confidence level.
Note that the Belle measurement has strongly non-Gaussian behaviour.
The interpretation of the
average given above has to be done with the greatest care.
We perform uncorrelated averages
(using the PDG prescription
for asymmetric errors).
Within the Standard Model, the b → s penguin transition carries approximately the same weak phase as the b → cc-bar s amplitude used above to obtain sin(2β) ≡ sin(2φ1). When this single phase dominates the decay to a (quasi-)two-body CP eigenstate, the time-dependent CP violation parameters should therefore by given by S = −ηCP × sin(2βeff) ≡ −ηCP × sin(2φ1eff) and C ≡ −A = 0. The loop process is sensitive to effects from virtual new physics particles, which may result in deviations from the prediction that sin(2βeff) ≡ sin(2φ1eff) (b → qq-bar s) ∼ sin(2β) ≡ sin(2φ1) (b → cc-bar s).
Various different final states have been used by BaBar and Belle to investigate time-dependent CP violation in hadronic b → s penguin transitions. These are summarised below. (Note that results from time-dependent Dalitz plot analyses of B0 → K+K−K0 and B0 → π+π−KS are also discussed in the next section — results for φK0, ρ0KS and f0KS are extracted from these analyses. The third error, where given, is due to Dalitz model uncertainty.)
At present we do not apply a rescaling of the results to a common, updated set of input parameters. We take correlations between S and C into account where available, except if one or more of the measurements suffers from strongly non-Gaussian errors. In that case, we perform uncorrelated averages (using the PDG prescription for asymmetric errors).
| Mode | Experiment | sin(2βeff) ≡ sin(2φ1eff) | CCP | Correlation | Reference |
|---|---|---|---|---|---|
| φK0 |
BaBar
(*)
N(BB)=470M |
0.66 ± 0.17 ± 0.07 | 0.05 ± 0.18 ± 0.05 | - | PRD 85 (2012) 112010 |
|
Belle
(*)
N(BB)=657M |
0.90 +0.09 −0.19 | −0.04 ± 0.20 ± 0.10 ± 0.02 | - | PRD 82 (2010) 073011 | |
| Average (*) | 0.74 +0.11 −0.13 | 0.01 ± 0.14 | - | HFAG | |
|
|
eps.gz png | eps.gz png | . | ||
| η′K0 |
BaBar
N(BB)=467M |
0.57 ± 0.08 ± 0.02 | −0.08 ± 0.06 ± 0.02 | 0.03 (stat) | PRD 79 (2009) 052003 |
|
Belle
N(BB)=535M |
0.64 ± 0.10 ± 0.04 | 0.01 ± 0.07 ± 0.05 | 0.09 (stat) | PRL 98 (2007) 031802 | |
| Average | 0.59 ± 0.07 | −0.05 ± 0.05 | 0.04 |
HFAG correlated average
χ2 = 0.9/2 dof (CL=0.63 ⇒ 0.5σ) |
|
|
|
eps.gz png | eps.gz png | eps.gz png | ||
| KSKSKS |
BaBar
N(BB)=468M |
0.94 +0.21 −0.24 ± 0.06 | −0.17 ± 0.18 ± 0.04 | 0.16 (stat) | PRD 85 (2012) 054023 |
|
Belle
N(BB)=535M |
0.30 ± 0.32 ± 0.08 | −0.31 ± 0.20 ± 0.07 | - | PRL 98 (2007) 031802 | |
| Average | 0.72 ± 0.19 | −0.24 ± 0.14 | 0.09 |
HFAG correlated average
χ2 = 2.7/2 dof (CL=0.26 ⇒ 1.1σ) |
|
|
|
eps.gz png | eps.gz png | eps.gz png | ||
| π0K0 |
BaBar
N(BB)=467M |
0.55 ± 0.20 ± 0.03 | 0.13 ± 0.13 ± 0.03 | 0.06 (stat) | PRD 79 (2009) 052003 |
|
Belle
N(BB)=657M |
0.67 ± 0.31 ± 0.08 | −0.14 ± 0.13 ± 0.06 | −0.04 (stat) | PRD 81 (2010) 011101 | |
| Average | 0.57 ± 0.17 | 0.01 ± 0.10 | 0.02 |
HFAG correlated average
χ2 = 2.0/2 dof (CL=0.37 ⇒ 0.9σ) |
|
|
|
eps.gz png | eps.gz png | eps.gz png | ||
| ρ0KS |
BaBar
(*)
N(BB)=383M |
0.35 +0.26 −0.31 ± 0.06 ± 0.03 | −0.05 ± 0.26 ± 0.10 ± 0.03 | - | PRD 80 (2009) 112001 |
|
Belle
(*)
N(BB)=657M |
0.64 +0.19 −0.25 ± 0.09 ± 0.10 | −0.03 +0.24 −0.23 ± 0.11 ± 0.10 | - | PRD 79 (2009) 072004 | |
| Average (*) | 0.54 +0.18 −0.21 | −0.06 ± 0.20 | - | HFAG | |
|
|
eps.gz png | eps.gz png | . | ||
| ωKS |
BaBar
N(BB)=467M |
0.55 +0.26 −0.29 ± 0.02 | −0.52 +0.22 −0.20 ± 0.03 | 0.03 (stat) | PRD 79 (2009) 052003 |
|
Belle
N(BB)=535M |
0.11 ± 0.46 ± 0.07 | 0.09 ± 0.29 ± 0.06 | −0.04 (stat) | PRD 76 (2007) 091103(R) | |
| Average | 0.45 ± 0.24 | −0.32 ± 0.17 | 0.01 |
HFAG correlated average
χ2 = 3.4/2 dof (CL=0.18 ⇒ 1.3σ) |
|
|
|
eps.gz png | eps.gz png | . | ||
| f0K0 | BaBar (**) | 0.74 +0.12 −0.15 | 0.15 ± 0.16 | - | HFAG (**) |
| Belle (**) | 0.63 +0.16 −0.19 | 0.13 ± 0.17 | - | HFAG (**) | |
| Average | 0.69 +0.10 −0.12 | 0.14 ± 0.12 | - | HFAG | |
|
|
eps.gz png | eps.gz png | . | ||
| f2KS |
BaBar
(*)
N(BB)=383M |
0.48 ± 0.52 ± 0.06 ± 0.10 | 0.28 +0.35 −0.40 ± 0.08 ± 0.07 | 0.01 (stat) | PRD 80 (2009) 112001 |
| fXKS |
BaBar
(*)
N(BB)=383M |
0.20 ± 0.52 ± 0.07 ± 0.07 | 0.13 +0.33 −0.35 ± 0.04 ± 0.09 | 0.29 (stat) | PRD 80 (2009) 112001 |
| π0π0KS (****) |
BaBar
N(BB)=227M |
−0.72 ± 0.71 ± 0.08 | 0.23 ± 0.52 ± 0.13 | −0.02 (stat) | PRD 76 (2007) 071101 |
| φ KS π0 |
BaBar
(***)
N(BB)=465M |
0.97 +0.03 −0.52 | −0.20 ± 0.14 ± 0.06 | - | PRD 78 (2008) 092008 |
| π+ π− KS nonresonant |
BaBar
(*)
N(BB)=383M |
0.01 ± 0.31 ± 0.05 ± 0.09 | 0.01 ± 0.25 ± 0.06 ± 0.05 | −0.11 (stat) | PRD 80 (2009) 112001 |
|
K+K−K0
(excluding φK0 and f0K0) |
BaBar
(*)
N(BB)=470M |
0.65 ± 0.12 ± 0.03 | 0.02 ± 0.09 ± 0.03 | - | PRD 85 (2012) 112010 |
|
Belle
(*)
N(BB)=657M |
0.76 +0.14 −0.18 | 0.14 ± 0.11 ± 0.08 ± 0.03 | - | PRD 82 (2010) 073011 | |
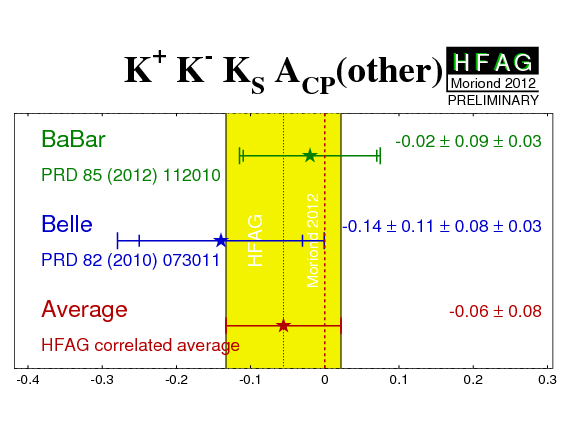
| Average | 0.68 +0.09 −0.10 |
0.06 ± 0.08
|
- | HFAG | |
|
|
eps.gz png | eps.gz png | . | ||
| Naïve b→s penguin average |
0.64 ± 0.03
χ2 = 19/24 dof (CL=0.74 ⇒ 0.3σ) |
−0.01 ± 0.03
χ2 = 19/24 dof (CL=0.74 ⇒ 0.3σ) |
uncorrelated averages | HFAG | |
| eps.gz png | eps.gz png | ||||
| Direct comparison of charmonium and s-penguin averages (see comments below): χ2 = 0.6 (CL=0.43 ⇒ 0.8σ) | |||||
(*)
BaBar and Belle results for φK0,
ρ0KS and
K+K−K0 (excluding φK0 and f0K0)
are determined from their
time-dependent Dalitz plot analyses of
B0 → K+K−K0 and
B0 → π+π−KS.
For the experimental results,
we quote Q2B parameters that are given in the respective references,
where possible.
(Belle have not reported Q2B S parameters from their
time-dependent Dalitz plot analysis of
B0 → K+K−KS,
so we convert their results on φ1.)
The averages of the directly fitted parameters
are more reliable than those of the Q2B parameters,
therefore we convert those results to give the averages quoted in the
table above.
BaBar results for f2KS,
fXKS and
π+ π− KS nonresonant
are determined from their
time-dependent Dalitz plot analysis of
B0 → π+π−KS.
(**) BaBar and Belle results for f0K0 are combinations of results from the two Dalitz plot analyses: B0 → f0K0 with f0 → K+K−, and B0 → f0KS with f0 → π+π−. Note that Q2B parameters extracted from Dalitz plot analyses are constrained to lie within the physical boundary (SCP2 + CCP2 < 1), and consequently the obtained errors can be highly non-Gaussian when the central value is close to the boundary. This is particularly evident in the BaBar results from B0 → f0KS with f0 → π+π−. These results must be treated with extreme caution. As above, we convert the averages of the directly fitted parameters from the time-dependent Dalitz plot analyses back to the Q2B parameters given in the table above.
(***) The BaBar results on φ KS π0 come from a simultaneous angular analysis of B → φ K+ π− and B → φ KS π0, where the angular parameters of the two decays modes are related since only (Kπ) resonances contribute to the final state. Note that Q2B parameters extracted in this way are constrained to lie within the physical boundary (SCP2 + CCP2 < 1), and consequently the obtained errors are highly non-Gaussian when the central value is close to the boundary. The single uncertainty given for sin(2βeff) in this result includes both statistical and systematic uncertainties.
(****) We do not include a preliminary result from Belle on π0π0KS that remains unpublished after more than two years.
Please note that
| Compilation of results for −η×S ≈ sin(2βeff) ≡ sin(2φ1eff) and C from s-penguin decays. |
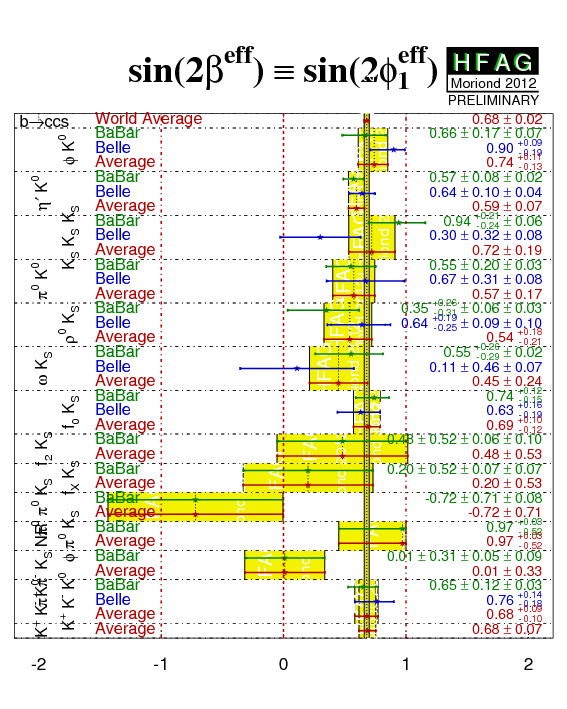
eps png |

eps png |
| Same, but without f2KS, fXKS π0π0KS, π+ π− KS nonresonant and φ KS π0 to allow closer inspection of the detail. |
|
|
| Comparisons of averages in the different b→q q-bar s modes |
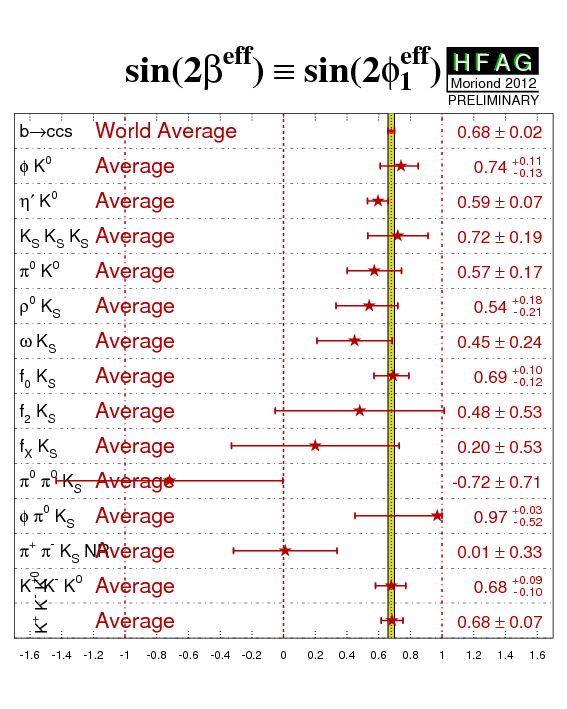
eps png |

eps png |
| Same, but without f2KS, fXKS π0π0KS, π+ π− KS nonresonant and φ KS π0 to allow closer inspection of the detail. |
|
|
| 2D comparisons of averages in the different b→q q-bar s modes. |
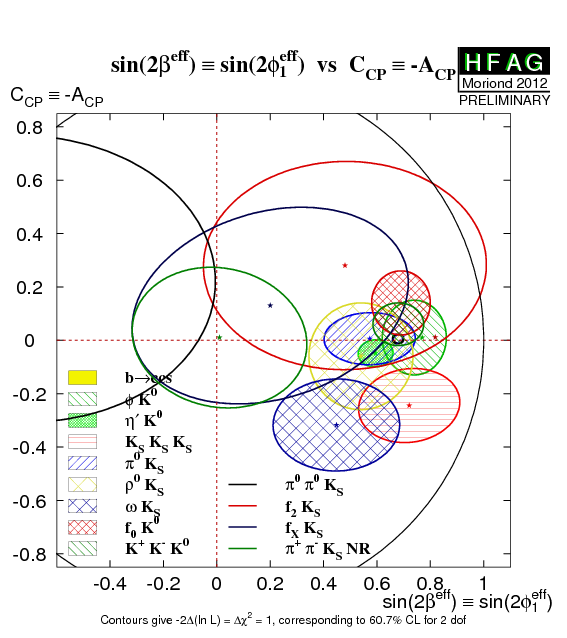
eps png |
|
Time-dependent amplitude analyses of the three-body decays Bd → K+K−K0 and Bd → π+π−K0 allow additional information to be extracted from the data. In particular, the cosine of the effective weak phase difference (cos(2βeff) ≡ cos(2φ1eff)) can be determined, as well as the sine term that is obtained from quasi-two-body analysis. This information allows half of the degenerate solutions to be rejected. Furthermore, Dalitz plot analysis has enhanced sensitivity to direct CP violation.
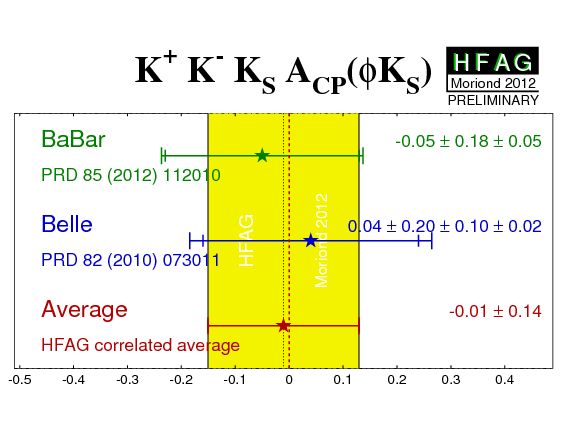
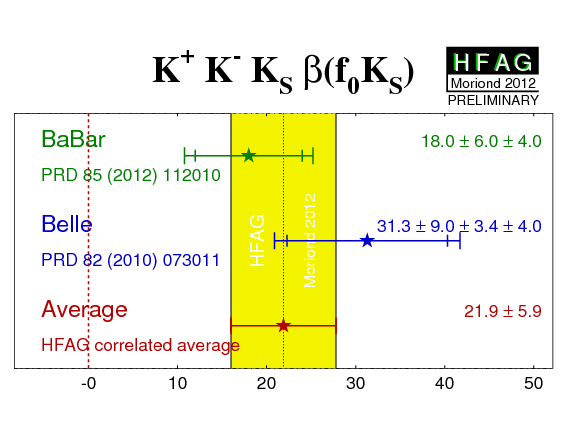
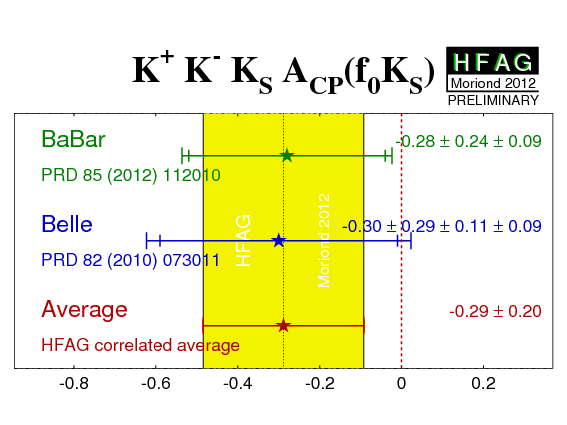
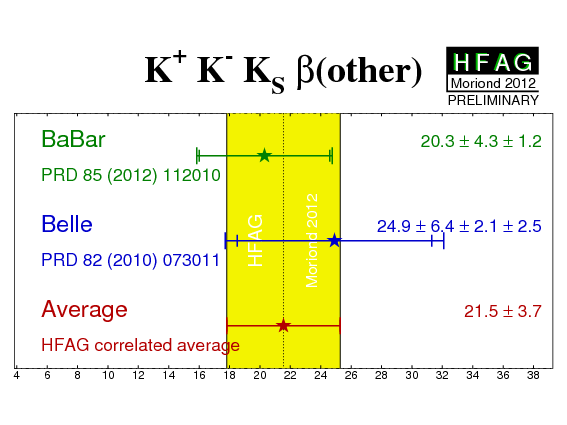
Time-dependent Dalitz plot analyses of B0 → K+K−KS have been performed by BaBar and Belle. As given above, parameters can be extracted in a form that allows a straightforward comparison/combination with those from time-dependent CP asymmetries in quasi-two-body b → qq-bar s modes. In addition, the effective weak phase βeff ≡ φ1eff is directly determined for two significant resonant contributions: φK0 and f0K0 and for the rest of the charmless contributions to the Dalitz plot combined, with the CP properties of the individual components taken into account.
| Experiment | φKS | f0KS | other K+K−KS | Correlation | Reference | |||
|---|---|---|---|---|---|---|---|---|
| βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | |||
|
BaBar
N(BB)=470M |
(21 ± 6 ± 2)° | −0.05 ± 0.18 ± 0.05 | (18 ± 6 ± 4)° | −0.28 ± 0.24 ± 0.09 | (20.3 ± 4.3 ± 1.2)° | −0.02 ± 0.09 ± 0.03 | (stat) | PRD 85 (2012) 112010 |
|
Belle
(*)
N(BB)=657M |
(32.2 ± 9.0 ± 2.6 ± 1.4)° | 0.04 ± 0.20 ± 0.10 ± 0.02 | (31.3 ± 9.0 ± 3.4 ± 4.0)° | −0.30 ± 0.29 ± 0.11 ± 0.09 | (24.9 ± 6.4 ± 2.1 ± 2.5)° | −0.14 ± 0.11 ± 0.08 ± 0.03 | (stat) | PRD 82 (2010) 073011 |
| Average | (24 ± 5)° | −0.01 ± 0.14 | (22 ± 6)° | −0.29 ± 0.20 | (21.6 ± 3.7)° | −0.06 ± 0.08 | (stat) |
HFAG correlated average
χ2 = 1.8/6 dof (CL=0.93 ⇒ 0.1σ) |
|
|
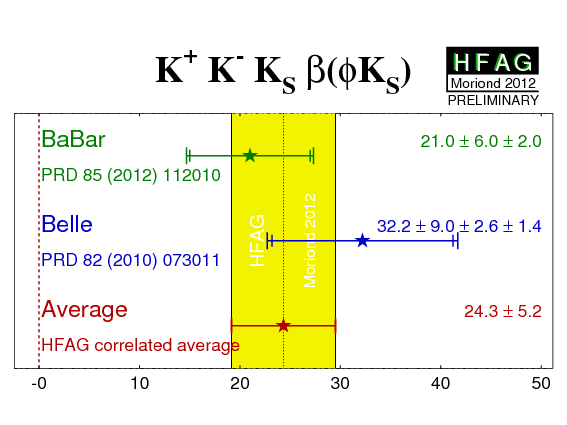
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
. | |
(*) The Belle results on φKS and f0KS suffer from a four-fold ambiguity in the solution. The results quoted here correspond to solution 1 presented in the paper. The third source of uncertainty arises due to the composition of the Dalitz plot.
From the above results BaBar infer that the trigonometric reflection at π/2 - &betaeff is disfavoured at 4.8σ.
Time-dependent Dalitz plot analyses of B0 → π+π−KS have been performed by BaBar and Belle. As given above, parameters can be extracted in a form that allows a straightforward comparison/combination with those from time-dependent CP asymmetries in quasi-two-body b → qq-bar s modes. In addition, the effective weak phase βeff ≡ φ1eff is directly determined for two significant resonant contributions: f0KS and ρ0KS by both experiments. Both experiments find multiple solutions in the fits; in both cases we quote the results given as solution 1. BaBar also report parameters related to the intermediate states f2(1270)KS, fX(1300)KS, nonresonant π+π−KS and χc0KS (see b → cc-bar s modes above). A number of additional parameters, for example relating to the Q2B modes K*+π−, are also extracted, but are not tabulated here.
The third error in the results given below is due to Dalitz model uncertainty.
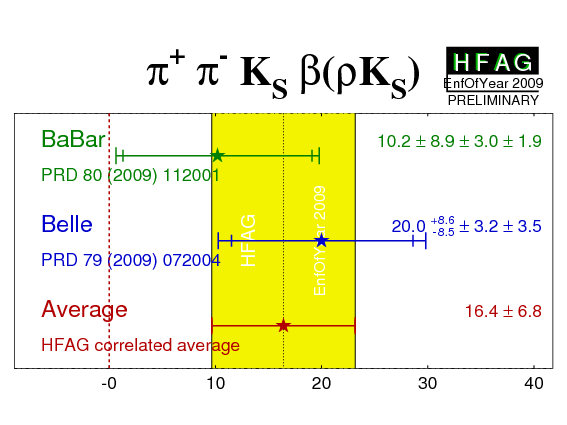
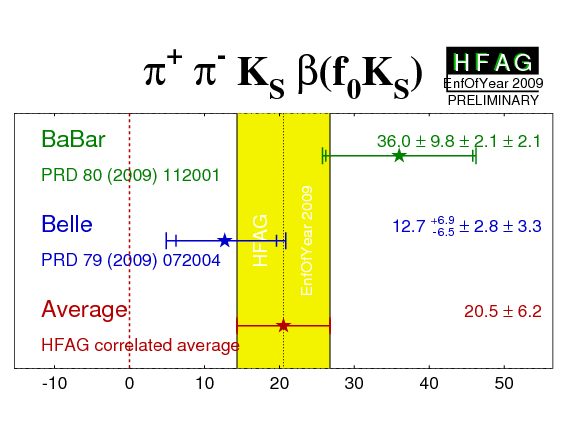
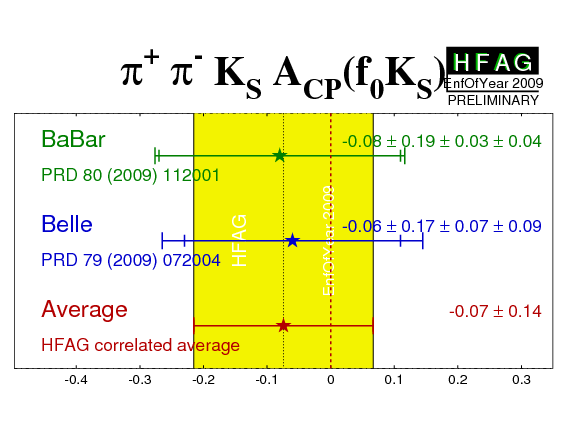
| Experiment | ρ0KS | f0KS | Correlation | Reference | ||
|---|---|---|---|---|---|---|
| βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | |||
|
BaBar
(*)
N(BB)=383M |
(10.2 ± 8.9 ± 3.0 ± 1.9)° | 0.05 ± 0.26 ± 0.10 ± 0.03 | (36.0 ± 9.8 ± 2.1 ± 2.1)° | −0.08 ± 0.19 ± 0.03 ± 0.04 | (stat) | PRD 80 (2009) 112001 |
|
Belle
(*)
N(BB)=657M |
(20.0 +8.6 −8.5 ± 3.2 ± 3.5)° | 0.03 +0.23 −0.24 ± 0.11 ± 0.10 | (12.7 +6.9 −6.5 ± 2.8 ± 3.3)° | −0.06 ± 0.17 ± 0.07 ± 0.09 | (stat) | PRD 79 (2009) 072004 |
| Average | (16.4 ± 6.8)° | 0.06 ± 0.20 | (20.6 ± 6.2)° | −0.07 ± 0.14 | (stat) |
HFAG correlated average
χ2 = 4.1/4 dof (CL=0.39 ⇒ 0.9σ) |
|
|
eps.gz png |

eps.gz png |
eps.gz png |
eps.gz png |
. | |
| Experiment | f2KS | fXKS | Nonresonant | χc0KS | Correlation | Reference | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | βeff ≡ φ1eff | ACP | |||
|
BaBar
(*)
N(BB)=383M |
(14.9 ± 17.9 ± 3.1 ± 5.2)° | −0.28 +0.40 −0.35 ± 0.08 ± 0.07 | (5.8 ± 15.2 ± 2.2 ± 2.3)° | −0.13 +0.35 −0.33 ± 0.04 ± 0.09 | (0.4 ± 8.8 ± 1.9 ± 3.8)° | −0.01 ± 0.25 ± 0.06 ± 0.05 | (23.2 ± 22.4 ± 2.3 ± 4.2)° | 0.29 +0.44 −0.53 ± 0.03 ± 0.05 | (stat) | PRD 80 (2009) 112001 |
(*) Both experiments suffer from ambiguities in the solutions. The results quoted here correspond to solution 1 presented in the papers.
Since parameters related to the B0 → f0KS decay are obtained in both B0 → K+K−K0 and B0 → π+π−KS, we show compilations and naïve (uncorrelated) averages below.
|
Naïve (uncorrelated) averages for f0KS parameters |
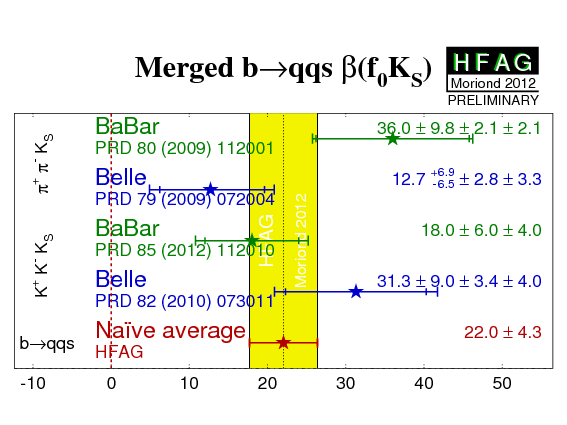
eps.gz png |

eps.gz png |
. | |
The final state in the decay B → φ KS π0 is a mixture of CP-even and CP-odd amplitudes. However, since only φ K*0 resonant states contribute (in particular, φ K*0(892), φ K*00(1430) and φ K*02(1430) are seen), the composition can be determined from the analysis of B → φ K+ π−, assuming only that the ratio of branching fractions B(K*0 → KS π0)/B(K*0 → K+ π−) is the same for each exited kaon state.
BaBar have performed a simultaneous analysis of B → φ KS π0 and B → φ K+ π− that is time-dependent for the former mode and time-integrated for the latter. Such an analysis allows, in principle, all parameters of the B → φ K*0 system to be determined, including mixing-induced CP violation effects. The latter is determined to be Δφ00 = 0.28 ± 0.42 ± 0.04, where Δφ00 is half the weak phase difference between B0 and B0-bar decays to φK*00(1430). As presented above, this can also be presented in terms of the quasi-two-body parameter sin(2βeff00) = sin(2β+2Δφ00) = 0.97 +0.03−0.52. The highly asymmetric uncertainty arises due to the conversion from the phase to the sine of the phase, and the proximity of the physical boundary.
Similar sin(2βeff) parameters can be defined for each of the helicity amplitudes for both φ K*0(892) and φ K*02(1430). However, the relative phases between these decays are constrained due to the nature of the simultaneous analysis of B → φ KS π0 and B → φ K+ π−, and therefore these measurements are highly correlated. Instead of quoting all these results, BaBar provide an illustration of their measurements with the following differences:
where the first subscript indicates the helicity amplitude and the second indicates the spin of the kaon resonance. For the complete definitions of the Δδ and Δφ parameters, please refer to the BaBar paper.
Direct CP violation parameters for each of the contributing helicity amplitudes can also be measured. Again, these are determined from a simultaneous fit of B → φ KS π0 and B → φ K+ π−, with the precision being dominated by the statistics of the latter mode. The direct CP violation measurements are tabulated by HFAG - Rare Decays.
The decay Bs → K+K− involves a b → uu-bar s transition, and hence has both penguin and tree contributions. Both mixing-induced and direct CP violation effects may arise, and additional input is needed to disentangle the contributions and determine γ and βseff. For example, the observables in Bd → π+π− can be related using U-spin, as proposed by Fleischer.
The observables are Amix = SCP, Adir = −CCP, and AΔΓ. They can all be treated as free parameters, but are physically constrained to satisfy Amix2 + Adir2 + AΔΓ2 = 1. Note that the untagged decay distribution, from which an "effective lifetime" can be measured, retains sensitivity to AΔΓ. Averages of effective lifetimes are performed by the HFAG lifetimes and oscillation group.
The observables have been measured by LHCb, who impose the constraint mentioned above to eliminate AΔΓ.
| Experiment | Amix | Adir | Correlation | Reference |
|---|---|---|---|---|
|
LHCb
∫Ldt=0.7 fb−1 |
0.17 ± 0.18 ± 0.05 | 0.02 ± 0.18 ± 0.04 | −0.10 (stat) | LHCb-CONF-2012-007 |
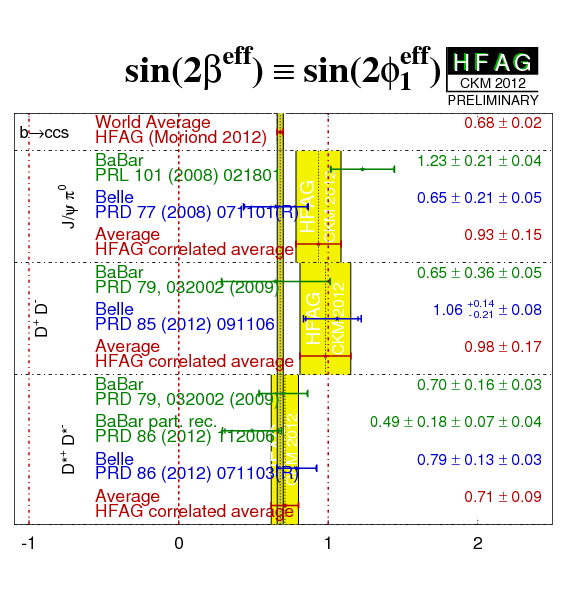
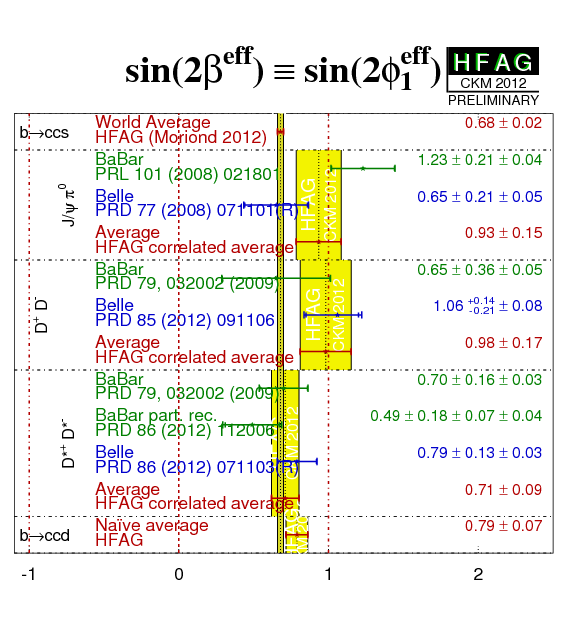
Due to possible significant penguin pollution, both the cosine and the sine coefficients of the Cabibbo-suppressed b → cc-bar d decays are free parameters of the theory. Absence of penguin pollution would result in Scc-bar d = − ηCP sin(2β) ≡ − ηCP sin(2φ1) and Ccc-bar d = 0 for the CP eigenstate final states (ηCP = +1 for both J/ψπ0 and D+D−).
For the non-CP eigenstates D*+−D−+, absence of penguin pollution (ie. no direct CP violation) gives A = 0, C+ = −C− (but is not necessarily zero), S+ = 2 R sin(2β+δ)/(1+R2) and S− = 2 R sin(2β−δ)/(1+R2). [With alternative notation, S+ = 2 R sin(2φ1+δ)/(1+R2) and S− = 2 R sin(2φ1−δ)/(1+R2)]. Here R is the ratio of the magnitudes of the amplitudes for B0 → D*+D− and B0 → D*−D+, while δ is the strong phase between them. If there is no CP violation of any kind, then S+ = −S− (but is not necessarily zero). An alternative notation, S = (S+ + S−)/2, Δ S = (S+ &minus S−)/2, C = (C+ + C−)/2, Δ C = (C+ &minus C−)/2, has been used in recent publications.
The vector-vector final state D*+D*− is a mixture of CP-even and CP-odd; the longitudinally polarized component is CP-even. Note that in the general case of non-negligible penguin contributions, the penguin-tree ratio and strong phase differences do not have to be the same for each helicity amplitude (likewise, they do not have to be the same for D*+D− and D*−D+).
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
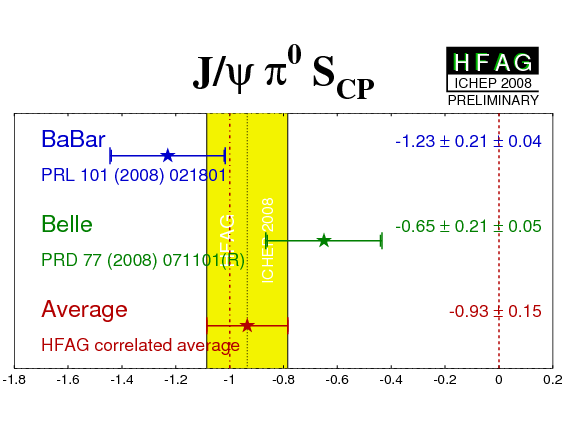
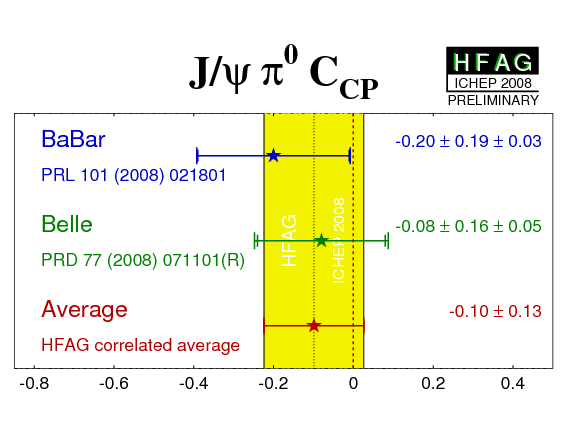
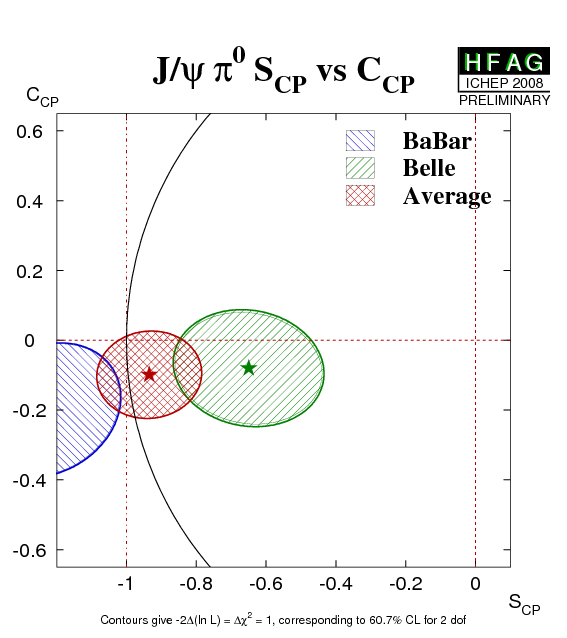
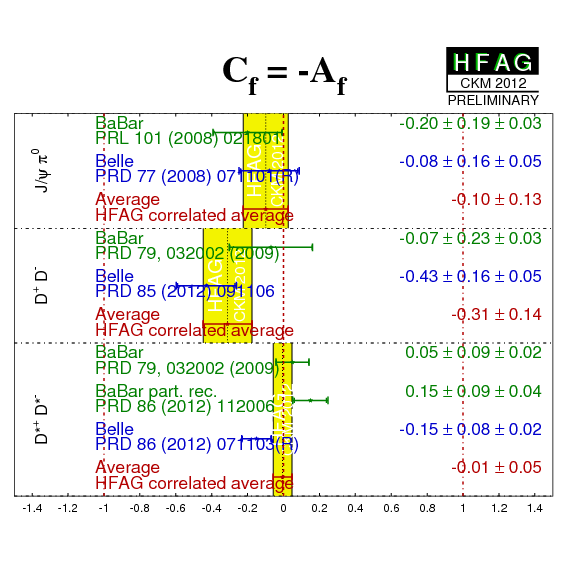
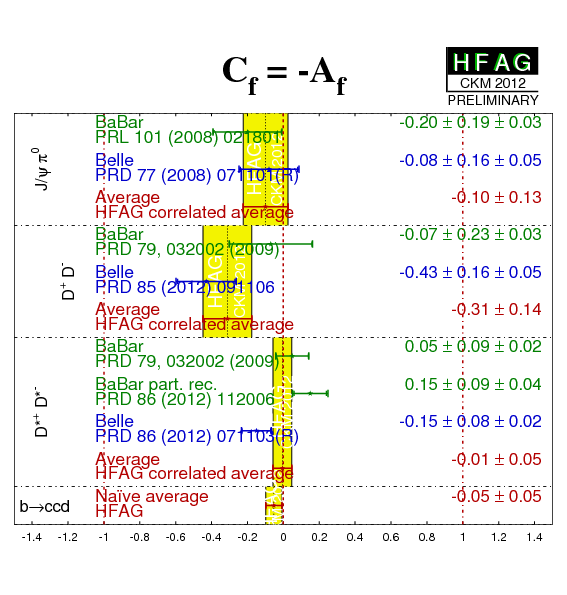
| Experiment | SCP (J/ψ π0) | CCP (J/ψ π0) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=466M |
−1.23 ± 0.21 ± 0.04 | −0.20 ± 0.19 ± 0.03 | 0.20 (stat) | PRL 101 (2008) 021801 |
|
Belle
N(BB)=535M |
−0.65 ± 0.21 ± 0.05 | −0.08 ± 0.16 ± 0.05 | −0.10 (stat) | PRD 77 (2008) 071101(R) |
| Average | −0.93 ± 0.15 | −0.10 ± 0.13 | 0.04 |
HFAG correlated average
χ2 = 3.8/2 dof (CL=0.15 ⇒ 1.4σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
(*) Note that the BaBar result is outside of the physical region, and the average is very close to the boundary. The interpretation of the average given above has to be done with the greatest care.
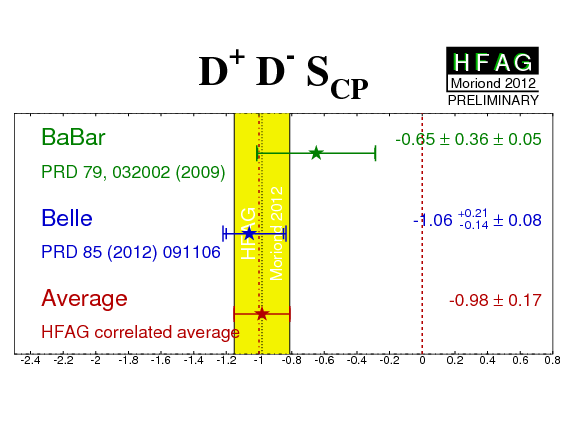
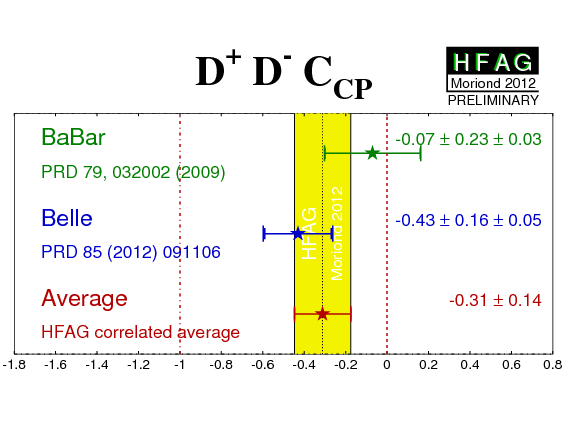
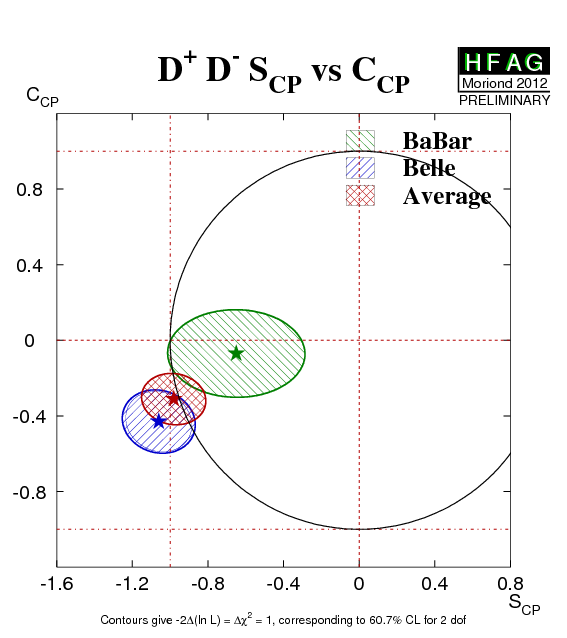
| Experiment | SCP (D+D−) | CCP (D+D−) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=467M |
−0.65 ± 0.36 ± 0.05 | −0.07 ± 0.23 ± 0.03 | −0.01 (stat) | PRD 79, 032002 (2009) |
|
Belle
N(BB)=772M |
−1.06 +0.21 −0.14 ± 0.08 | −0.43 ± 0.16 ± 0.05 | −0.12 (stat) | PRD 85 (2012) 091106 |
| Average | −0.98 ± 0.17 | −0.31 ± 0.14 | −0.08 |
HFAG correlated average
χ2 = 2.7/2 dof (CL=0.26 ⇒ 1.1σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
(*) Note that the Belle result is outside of the physical region, and the average is very close to the boundary. The interpretation of the average given above has to be done with the greatest care.
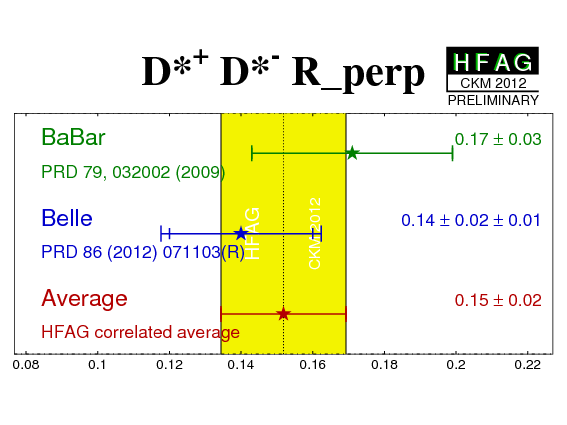
The vector particles in the pseudoscalar to vector-vector decay Bd → D*+D*− can have longitudinal and transverse relative polarization with different CP properties. The transversely polarized state (h⊥) is CP-odd, while the other two states in the transversity basis (h0 and h||) are CP-even. The CP parameters therefore have an important dependence on the fraction of the transversely polarized component R⊥.
In the most recent results, Belle performs an initial fit to determine the transversely polarized fraction R⊥, and then include effects due to its uncertainty together with other systematic errors. (In the most recent update Belle include R⊥ and also R0 as free parameters in the fit. We do not include information on R0 in the average for now.) BaBar treat R⊥ as a free parameter in the fit and consequently this systematic is absorbed in the statistical error. We perform the average taking into account correlations of the CP parameters with each other as well as with R⊥.
Belle have performed a fit to the data assuming that the CP parameters for CP-even and CP-odd transversity states are the same (up to a trivial change of sign for SCP). BaBar have performed two fits to the data: in addition to a fit as above, an additional fit relaxes this assumption, so that differences between CP-even and CP-odd parameters may be nontrivial. We use the first set of results to perform an average with Belle, and tabulate also the latter set of results. We also include the results of a separate analysis from BaBar based on partially reconstructed D*D* decays; in this analysis the BaBar measurement of R⊥ is used to correct the value of S fitted without separating CP-even and -odd components for the CP-odd dilution.
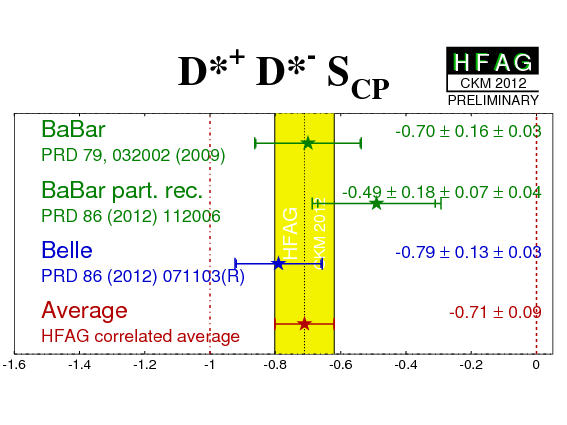
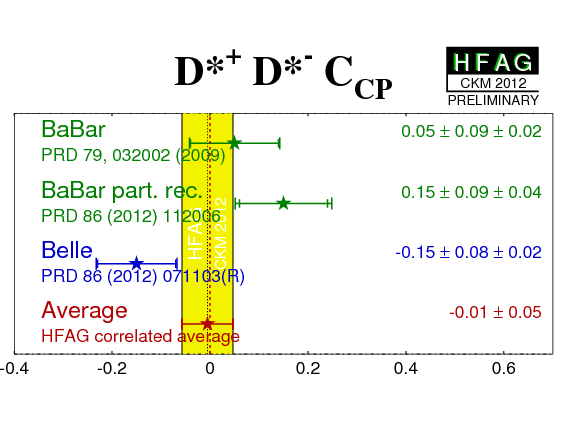
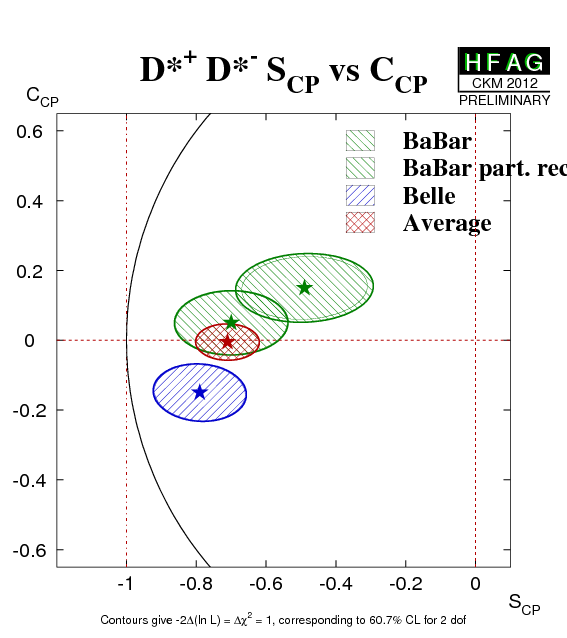
| Experiment | SCP (D*+ D*−) | CCP (D*+ D*−) | R⊥ (D*+ D*−) | Correlation | Reference |
|---|---|---|---|---|---|
|
BaBar
N(BB)=467M |
−0.70 ± 0.16 ± 0.03 | 0.05 ± 0.09 ± 0.02 | 0.17 ± 0.03 | (stat) | PRD 79, 032002 (2009) |
|
BaBar part. rec.
N(BB)=471M |
−0.49 ± 0.18 ± 0.07 ± 0.04 | 0.15 ± 0.09 ± 0.04 | - | (stat) | PRD 86 (2012) 112006 |
|
Belle
N(BB)=772M |
−0.79 ± 0.13 ± 0.03 | −0.15 ± 0.08 ± 0.02 | 0.14 ± 0.02 ± 0.01 | (stat) | PRD 86 (2012) 071103(R) |
| Average | −0.71 ± 0.09 | −0.01 ± 0.05 | 0.15 ± 0.02 | (stat) |
HFAG correlated average
χ2 = 3.7/6 dof (CL=0.72 ⇒ 0.4σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
|
| Experiment | S+ (D*+ D*−) | C+ (D*+ D*−) | S− (D*+ D*−) | C− (D*+ D*−) | R⊥ (D*+ D*−) | Correlation | Reference |
|---|---|---|---|---|---|---|---|
|
BaBar
N(BB)=467M |
−0.76 ± 0.16 ± 0.04 | 0.02 ± 0.12 ± 0.02 | −1.81 ± 0.71 ± 0.16 | 0.41 ± 0.50 ± 0.08 | 0.15 ± 0.03 | (stat) | PRD 79, 032002 (2009) |
(*) Note that the BaBar values of R⊥ in these tables are not corrected for efficiency; the efficiency corrected value is R⊥ = 0.158 ± 0.028 ± 0.006.
| Experiment | S(D*+D−) | C(D*+D−) | ΔS(D*−D+) | ΔC(D*−D+) | A(D*+−D−+) | Reference | |
|---|---|---|---|---|---|---|---|
|
BaBar
N(BB)=467M |
−0.68 ± 0.15 ± 0.04 | 0.04 ± 0.12 ± 0.03 | 0.05 ± 0.15 ± 0.02 | 0.04 ± 0.12 ± 0.03 | 0.01 ± 0.05 ± 0.01 | PRD 79, 032002 (2009) | |
|
Belle
N(BB)=772M |
−0.78 ± 0.15 ± 0.05 | −0.01 ± 0.11 ± 0.04 | −0.13 ± 0.15 ± 0.04 | 0.12 ± 0.11 ± 0.03 | 0.06 ± 0.05 ± 0.02 | PRD 85 (2012) 091106 | |
| Average |
−0.73 ± 0.11
χ2 = 0.20 (CL=0.65 ⇒ 0.5σ) |
0.01 ± 0.09
χ2 = 0.1 (CL=0.77 ⇒ 0.3σ) |
−0.04 ± 0.11
χ2 = 0.7 (CL=0.41 ⇒ 0.8σ) |
0.08 ± 0.08
χ2 = 0.2 (CL=0.63 ⇒ 0.5σ) |
0.03 ± 0.04
χ2 = 0.5 (CL=0.48 ⇒ 0.7σ) |
HFAG uncorrelated averages |
|
|
|
eps.gz png | eps.gz png | eps.gz png | eps.gz png | eps.gz png | . | |
| Compilation of results for (left) sin(2βeff) ≡ sin(2φ1eff) = −ηCPS and (right) C ≡ −A from time-dependent b → cc-bar d analyses with CP eigenstate final states. The results are compared to the values from the corresponding charmonium averages. |
eps.gz png |
eps.gz png |
||||
| Same, but with separate CP-even and CP-odd results from D*+D*− |
|
|
||||
|
Same, but including results from
D*+−D−+.
(These measure the same quantity as other b → cc-bar d modes when the strong phase difference between the two decay amplitudes vanishes. This is in addition to the usual assumption of negligible penguin contributions.) |
|
|
||||
Same, but including a naïve b → c c-bar d average.
Such an average assumes that penguin contributions to the
b → c c-bar d decays are negligible.
See the cautionary comments in the discussion on averaging
the time-dependent CP violation parameters for
b → qq-bar s transitions above.
The results of the naïve average are
|
eps.gz png |
eps.gz png |
||||
| 2D comparisons of averages in the different b→c c-bar d modes. |
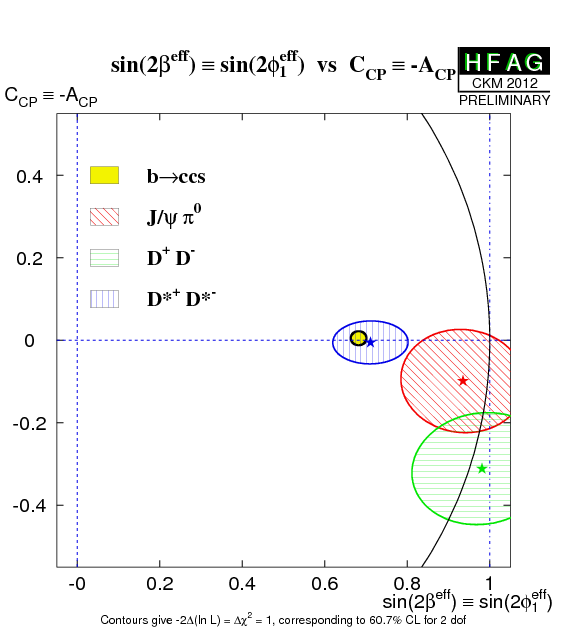
eps png |
|||||
The b → qq-bar d penguin transitions are suppressed in the Standard Model, leading to small numbers of events available in these final states. If the top quark dominates in the loop, the phase in the decay amplitude (β ≡ φ1) cancels that in the B0–B0-bar mixing, so that S = C = 0. However, even within the Standard Model, there may be non-negligible contributions with u or c quarks in the penguin loop (or from rescattering, etc.) so that different values of S and C are possible. In this case, these can be used to obtain constraints on γ ≡ φ3, and hence test if any non-Standard Model contributions are present.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
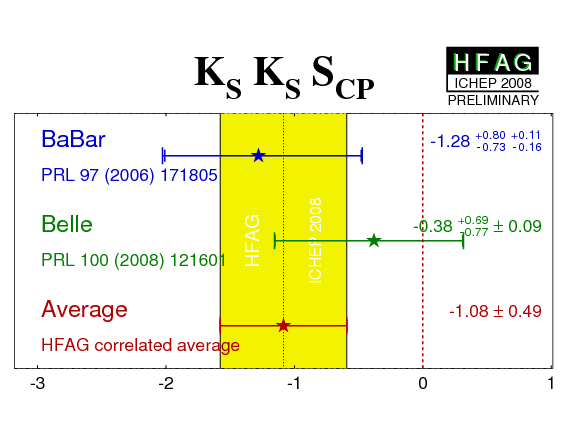
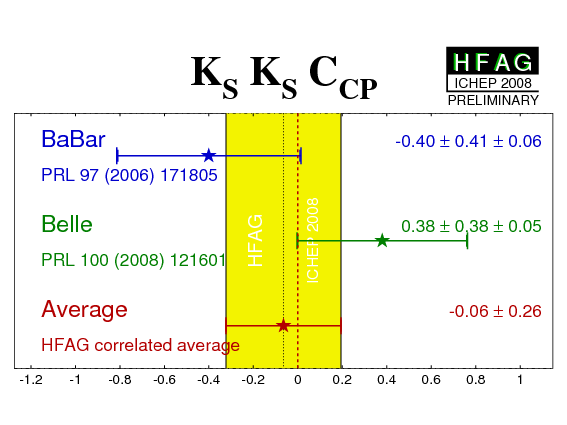
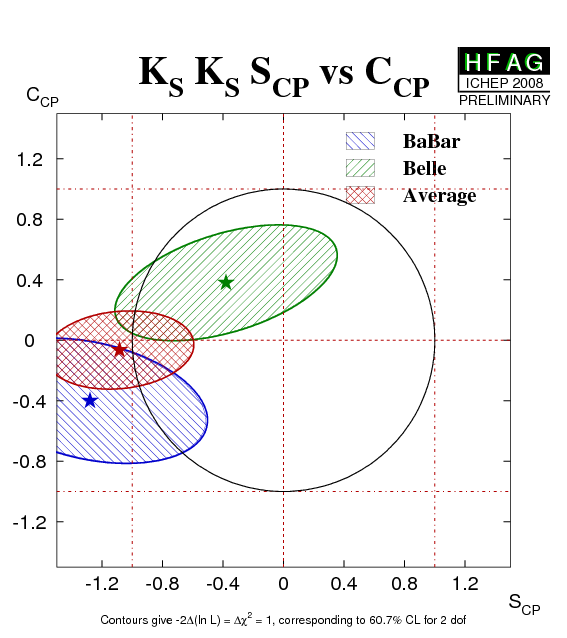
| Experiment | SCP (KSKS) | CCP (KSKS) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=350M |
−1.28 +0.80 −0.73 +0.11 −0.16 | −0.40 ± 0.41 ± 0.06 | −0.32 (stat) | PRL 97 (2006) 171805 |
|
Belle
N(BB)=657M |
−0.38 +0.69 −0.77 ± 0.09 | 0.38 ± 0.38 ± 0.05 | 0.48 (stat) | PRL 100 (2008) 121601 |
| Average | −1.08 ± 0.49 | −0.06 ± 0.26 | 0.14 |
HFAG correlated average
χ2 = 2.5/2 dof (CL=0.29 ⇒ 1.1σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
(*) Note that the BaBar result is outside of the physical region, as is the average. The interpretation of the results given above has to be done with the greatest care.
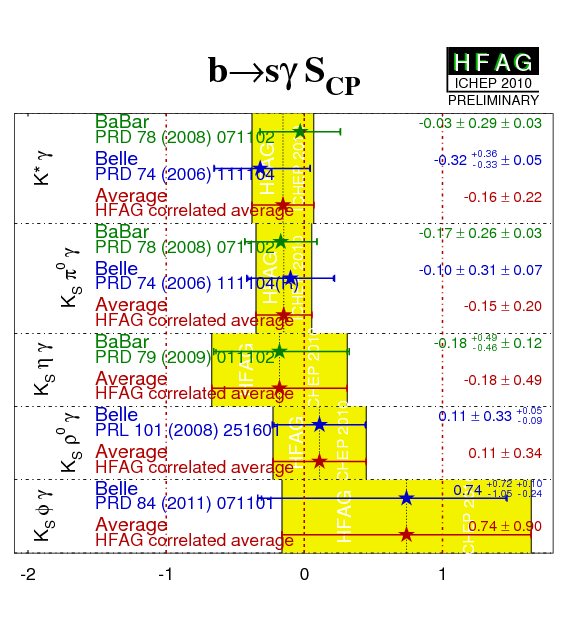
Time-dependent analyses of radiative b decays such as B0→ KSπ0γ, probe the polarization of the photon. In the SM, the photon helicity is dominantly left-handed for b → sγ, and right-handed for the conjugate process. As a consequence, B0 → KSπ0γ behaves like an effective flavor eigenstate, and mixing-induced CP violation is expected to be small - a simple estimation gives: S ~ −2(ms/mb)sin(2β) ≡ −2(ms/mb)sin(2φ1) (with an assumption that the Standard Model dipole operator is dominant). Corrections to the above may allow values of S as large as 10% in the SM.
Atwood et al. have shown that (with the same assumption) an inclusive analysis with respect to KSπ0 can be performed, since the properties of the decay amplitudes are independent of the angular momentum of the KSπ0 system. However, if non-dipole operators contribute significantly to the amplitudes, then the Standard Model mixing-induced CP violation could be larger than the expectation given above, and the CPV parameters may vary slightly over the KSπ0γ Dalitz plot, for example as a function of the KSπ0 invariant mass.
An inclusive KSπ0γ analysis has been performed by Belle using the invariant mass range up to 1.8 GeV/c2. Belle also gives results for the K*(892) region: 0.8 GeV/c2 to 1.0 GeV/c2. BABAR has measured the CP-violating asymmetries separately within and outside the K*(892) mass range: 0.8 GeV/c2 to 1.0 GeV/c2 is again used for K*(892)γ candidates, while events with invariant masses in the range 1.1 GeV/c2 to 1.8 GeV/c2 are used in the "KSπ0γ (not K*(892)γ)" analysis.
We quote two averages: one for K*(892) only, and the other one for the inclusive KSπ0γ decay (including the K*(892)). If the Standard Model dipole operator is dominant, both should give the same quantities (the latter naturally with smaller statistical error). If not, care needs to be taken in interpretation of the inclusive parameters; while the results on the K*(892) resonance remain relatively clean.
In addition to results with the KSπ0γ final state, BABAR has results using KSηγ, while Belle has results using KSρ0γ and using KSφ0γ.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
| Mode | Experiment | SCP (b → sγ) | CCP (b → sγ) | Correlation | Reference | ||
|---|---|---|---|---|---|---|---|
| K*(892)γ |
BaBar
N(BB)=467M |
−0.03 ± 0.29 ± 0.03 | −0.14 ± 0.16 ± 0.03 | 0.05 (stat) | PRD 78 (2008) 071102 | ||
|
Belle
N(BB)=535M |
−0.32 +0.36 −0.33 ± 0.05 | 0.20 ± 0.24 ± 0.05 | 0.08 (stat) | PRD 74 (2006) 111104 | |||
| Average | −0.16 ± 0.22 | −0.04 ± 0.14 | 0.06 |
HFAG correlated average
χ2 = 1.9/2 dof (CL=0.40 ⇒ 0.9σ) |
|||
|
KSπ0γ
(incl. K*γ) |
BaBar
N(BB)=467M |
−0.17 ± 0.26 ± 0.03 | −0.19 ± 0.14 ± 0.03 | 0.04 (stat) | PRD 78 (2008) 071102 | ||
|
Belle
N(BB)=535M |
−0.10 ± 0.31 ± 0.07 | 0.20 ± 0.20 ± 0.06 | 0.08 (stat) | PRD 74 (2006) 111104(R) | |||
| Average | −0.15 ± 0.20 | −0.07 ± 0.12 | 0.05 |
HFAG correlated average
χ2 = 2.4/2 dof (CL=0.30 ⇒ 1.0σ) |
|||
| KS η γ |
BaBar
N(BB)=465M |
−0.18 +0.49 −0.46 ± 0.12 | −0.32 +0.40 −0.39 ± 0.07 | −0.17 (stat) | PRD 79 (2009) 011102 | ||
| KS ρ0 γ |
Belle
N(BB)=657M |
0.11 ± 0.33 +0.05 −0.09 | −0.05 ± 0.18 ± 0.06 | 0.04 (stat) | PRL 101 (2008) 251601 | ||
| KS φ γ |
Belle
N(BB)=772M |
0.74 +0.72 −1.05 +0.10 −0.24 | −0.35 ± 0.58 +0.10 −0.23 | - | PRD 84 (2011) 071101 | ||
|
|
eps.gz png |

eps.gz png |
|
||||
Similar to the b → sγ transitions discussed above, time-dependent analyses of radiative b decays such as B0→ ρ0γ probe the polarization of the photon emitted in radiative b → dγ decays. However, since the CP violating phase from the b → d decay amplitude cancels that from the Bd–Bd-bar mixing (to an approximation that is exact in the limit of top quark dominance in the loops), the asymmetry is suppressed even further in the Standard Model. An observable signal would be a sign of a new physics amplitude emitting right-handed photons and carrying a new CP violating phase.
A time-dependent analysis of the B0→ ρ0γ channel has been carried out by Belle.
At present we do not apply a rescaling of the results to a common, updated set of input parameters.
| Experiment | SCP (b → dγ) | CCP (b → dγ) | Correlation | Reference |
|---|---|---|---|---|
|
Belle
N(BB)=657M |
−0.83 ± 0.65 ± 0.18 | 0.44 ± 0.49 ± 0.14 | −0.08 (stat) | PRL 100 (2008) 021602 |
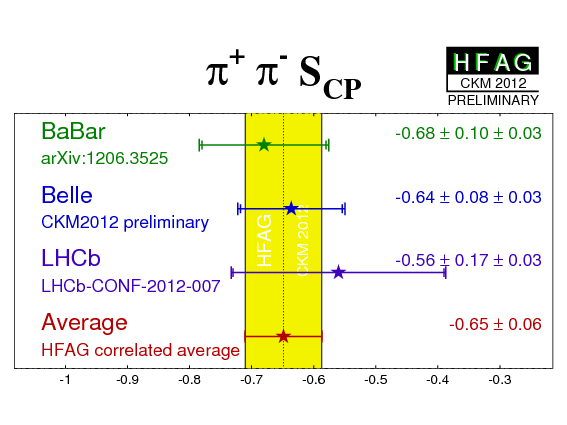
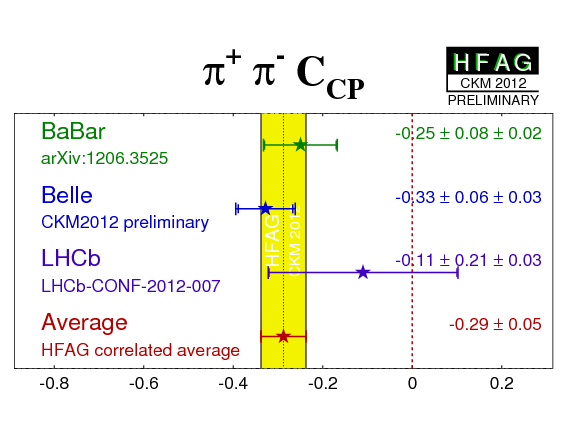
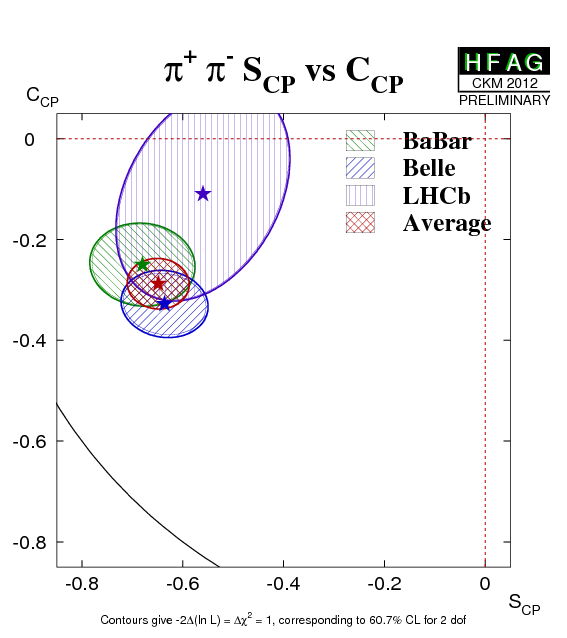
The observables have been measured by BaBar, Belle & LHCb. Note that at the B factories the observables are in principle uncorrelated (due to the fact that the time variable, Δt, has the range −∞ < Δt < +∞ – small correlations can be induced e.g.by backgrounds), at hadron colliders a non-zero correlation is expected (the time variable t takes the range 0 < t < +∞). Please note that at present we do not apply a rescaling of the results to a common, updated set of input parameters. Correlation due to common systematics are neglected in the following averages. We recall that we do NOT rescale (inflate) the errors due to measurement inconsistencies.
| Experiment | SCP (π+π−) | CCP (π+π−) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=467M |
−0.68 ± 0.10 ± 0.03 | −0.25 ± 0.08 ± 0.02 | −0.06 (stat) | arXiv:1206.3525 |
|
Belle
N(BB)=772M |
−0.64 ± 0.08 ± 0.03 | −0.33 ± 0.06 ± 0.03 | −0.10 (stat) | CKM2012 preliminary |
|
LHCb
∫Ldt=0.7 fb−1 |
−0.56 ± 0.17 ± 0.03 | −0.11 ± 0.21 ± 0.03 | 0.34 (stat) | LHCb-CONF-2012-007 |
| Average | −0.65 ± 0.06 | −0.29 ± 0.05 | −0.03 |
HFAG correlated average
χ2 = 1.4/4 dof (CL=0.84 ⇒ 0.2σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
Interpretations:
The
Gronau-London
isospin analysis allows a constraint on α ≡ φ2
to be extracted from the ππ system even in the presence of non-negligible
penguin contributions.
The analysis involves the SU(2) partners of
the Bd→ π+π− decay.
The relevant branching ratios (given in units of 10−6)
and CP-violating charge asymmetries are taken from
HFAG - Rare Decays.
| BR(B0 → π+π−) = 5.16 ± 0.22 | - |
| BR(B+ → π+π0) = 5.59 ±0.41 | ACP(B+ → π+π0) = 0.06 ± 0.05 |
| BR(B0 → π0π0) = 1.55 ± 0.19 | ACP(B0 → π0π0) = 0.43 +0.25−0.24 |
Belle
exclude the range 9° < φ2 < 81° at the 95.4% confidence level.
BaBar
give a confidence level interpretation for α
and exclude the range 23° < α < 67° at the 90% confidence level.
NB. It is implied in the above constraints on α ≡ φ2 that a mirror solution at α → α + π ≡ φ2 → φ2 + π also exists.
For more details on the world average for α ≡ φ2, calculated with different statistical treatments, refer to the CKMfitter and UTfit pages.
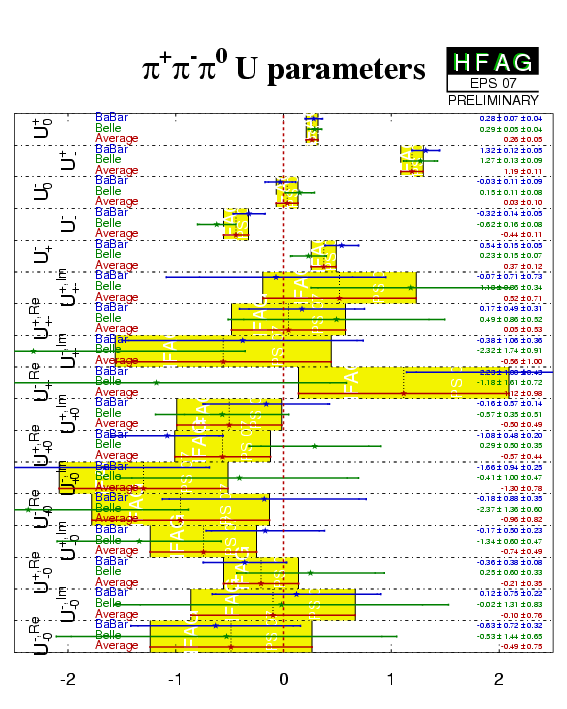
Both BaBar and Belle have performed a full time-dependent Dalitz plot analyses of the decay Bd → (ρπ)0 → π+π−π0, which allows to simultaneously determine the complex decay amplitudes and the CP-violating weak phase α ≡ φ2. The analysis follows the idea of Snyder and Quinn (1993), implemented as suggested by Quinn and Silva. The experiments determine 27 coefficients of the form factor bilinears from the fit to data. Physics parameters, such as the quasi-two-body parameters, and the phases δ+−=arg[A−+A+−*] and the UT angle α ≡ φ2, are determined from subsequent fits to the bilinear coefficients.
Please note that at present we do not apply a rescaling of the results to a common, updated set of input parameters. Correlation due to common systematics are neglected in the following averages.
| [The table of averages of the form factor bilinears is suppressed here for the benefit of the nonspecialist. Those interested in the details can find them here.] |
| Compilation of averages of the form factor bilinears. |
eps.gz png |
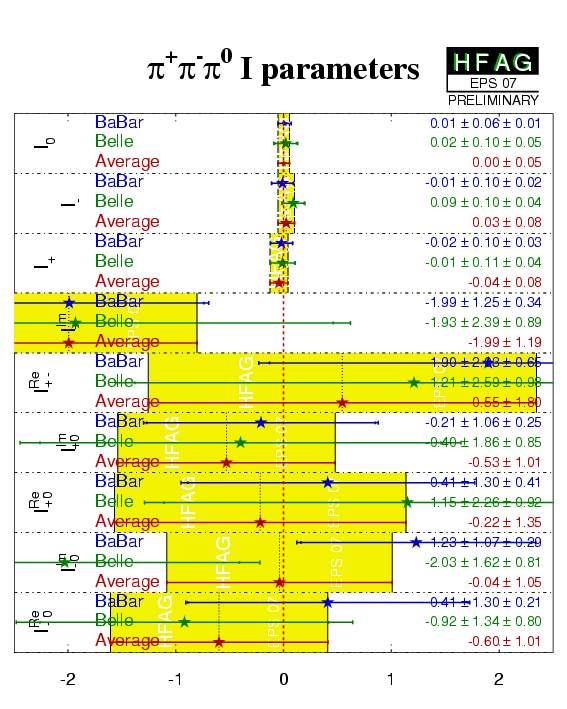
eps.gz png |
From the bilinear coefficients given above, both experiments extract "quasi-two-body" (Q2B) parameters. Considering only the charged ρ bands in the Dalitz plot, the Q2B analysis involves 5 different parameters, one of which − the charge asymmetry ACP(ρπ) − is time-independent. The time-dependent decay rate is given by
where Qtag=+1(−1) when the tagging meson is a B0 (B0-bar). CP symmetry is violated if either one of the following conditions is true: ACP(ρπ)≠0, Cρπ≠0 or Sρπ≠0. The first two correspond to CP violation in the decay, while the last condition is CP violation in the interference of decay amplitudes with and without Bd mixing.
We average the quasi-two-body parameters provided by the experiments, which should be equivalent to determining average values directly from the averaged bilinear coefficients.
As shown by
Charles
it can be convenient to transform the experimentally motivated
CP parameters ACP(ρπ) and Cρπ
into the physically motivated choices
A+−(ρπ) =
(|κ+−|2−1)/(|κ+−|2+1) =
−(ACP(ρπ)+Cρπ+ACP(ρπ)ΔCρπ)/(1+ΔCρπ + ACP(ρπ)Cρπ),
A−+(ρπ) =
(|κ−+|2−1)/(|κ−+|2+1) =
(−ACP(ρπ)+Cρπ+ACP(ρπ)ΔCρπ)/(−1+ΔCρπ + ACP(ρπ)Cρπ),
where
κ+− = (q/p)Abar−+/A+− and
κ−+ = (q/p)Abar+−/A−+.
With this definition A−+(ρπ) (A+−(ρπ))
describes CP violation in Bd decays
where the ρ is emitted (not emitted) by the spectator interaction.
Both experiments obtain values for A+− and A−+,
which we average.
Again, this procedure should be equivalent to extracting these values
directly from the previous results.
In addition to the Bd→ ρ+−π−+ quasi-two-body contributions to the π+π−π0 final state, there can also be a Bd→ ρ0π0 component. Both experiments have also extracted the quasi-two-body parameters associated with this intermediate state.
Note again that at present we do not apply a rescaling of the results to a common, updated set of input parameters. Correlations due to possible common systematics are neglected in the following averages.
The citation given for Belle in the tables below corresponds to a short article published in PRL. A more detailed article on the same analysis is also available as arXiv:0710.4974 [hep-ex].
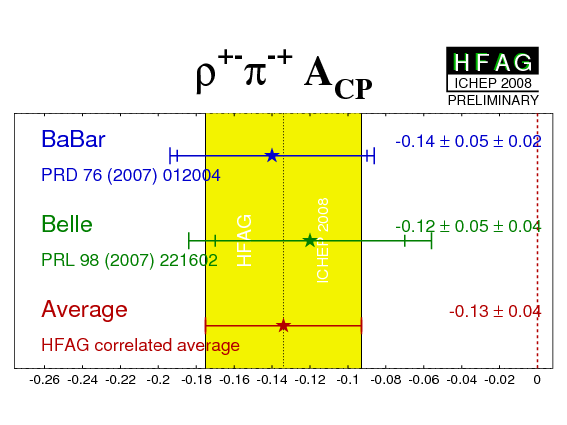
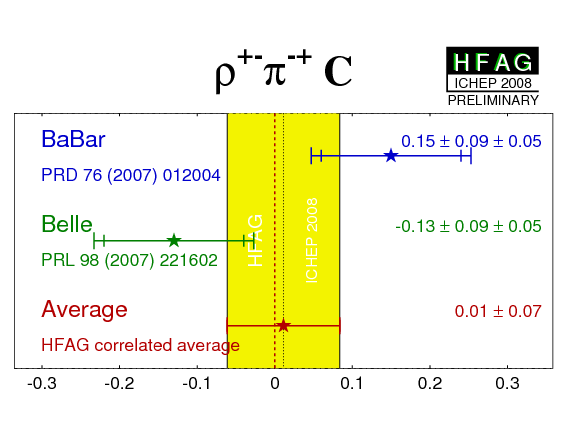
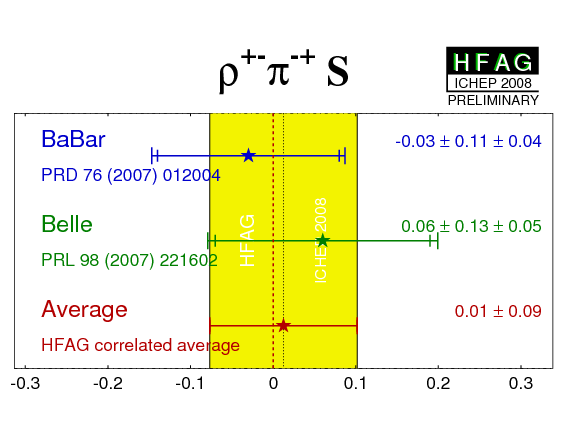
| Experiment | ACP (ρ+−π−+) | C (ρ+−π−+) | S (ρ+−π−+) | ΔC (ρ+−π−+) | ΔS (ρ+−π−+) | Correlations | Reference |
|---|---|---|---|---|---|---|---|
|
BaBar
N(BB)=375M |
−0.14 ± 0.05 ± 0.02 | 0.15 ± 0.09 ± 0.05 | −0.03 ± 0.11 ± 0.04 | 0.39 ± 0.09 ± 0.09 | −0.01 ± 0.14 ± 0.06 | (stat) | PRD 76 (2007) 012004 |
|
Belle
N(BB)=449M |
−0.12 ± 0.05 ± 0.04 | −0.13 ± 0.09 ± 0.05 | 0.06 ± 0.13 ± 0.05 | 0.36 ± 0.10 ± 0.05 | −0.08 ± 0.13 ± 0.05 | (stat) | PRL 98 (2007) 221602 |
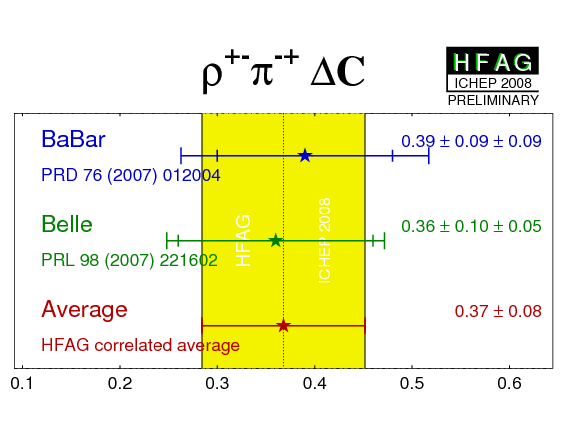
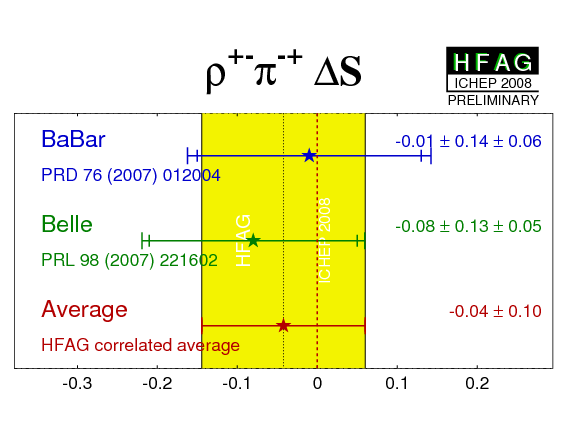
| Average | −0.13 ± 0.04 | 0.01 ± 0.07 | 0.01 ± 0.09 | 0.37 ± 0.08 | −0.04 ± 0.10 | (stat) |
HFAG correlated average
χ2 = 4.2/5 dof (CL=0.52 ⇒ 0.6σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
eps.gz png |
. | |
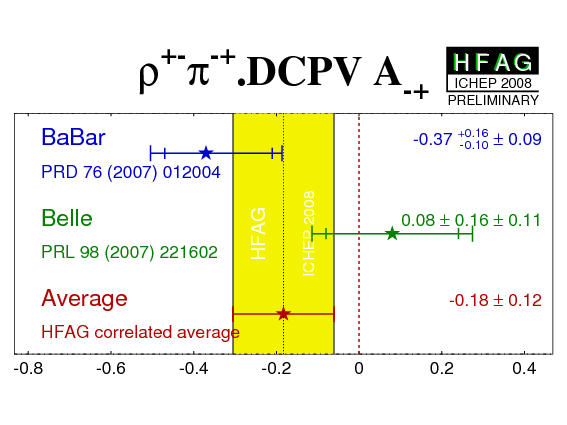
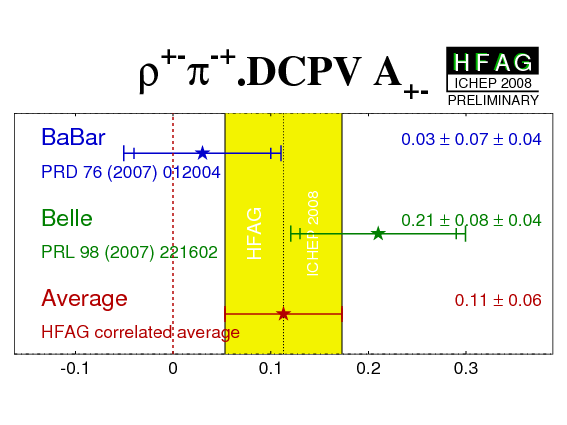
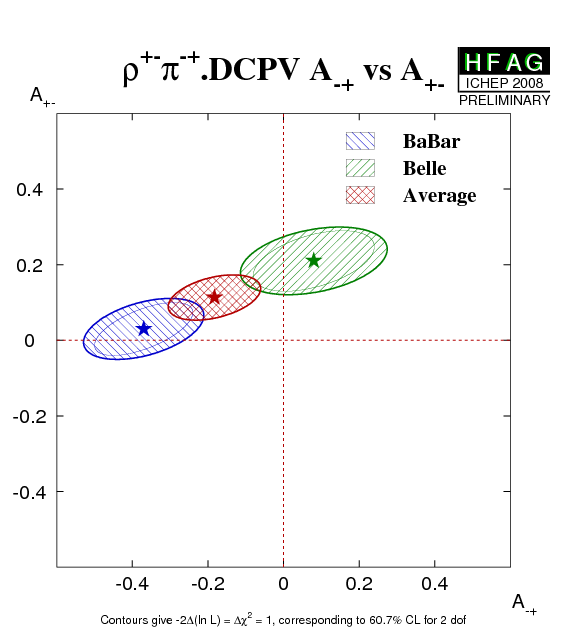
| Experiment | A−+ (ρ+−π−+) | A+− (ρ+−π−+) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=375M |
−0.37 +0.16 −0.10 ± 0.09 | 0.03 ± 0.07 ± 0.04 | 0.62 | PRD 76 (2007) 012004 |
|
Belle
N(BB)=449M |
0.08 ± 0.16 ± 0.11 | 0.21 ± 0.08 ± 0.04 | 0.47 | PRL 98 (2007) 221602 |
| Average | −0.18 ± 0.12 | 0.11 ± 0.06 | 0.40 |
HFAG correlated average
χ2 = 4.0/2 dof (CL=0.14 ⇒ 1.5σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
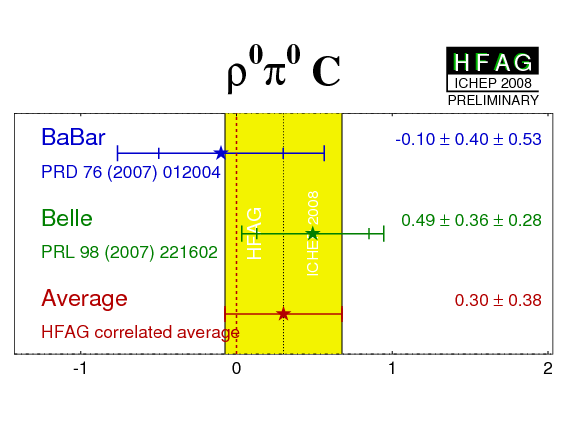
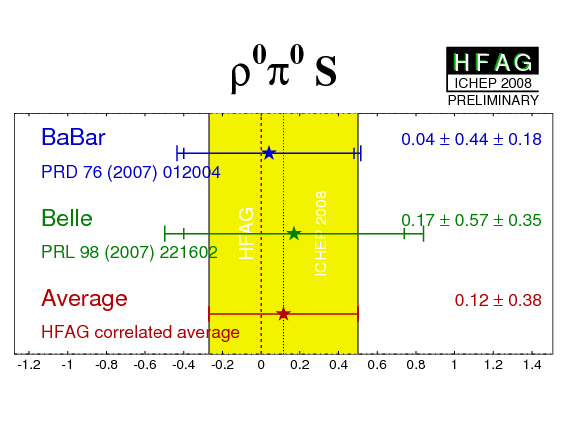
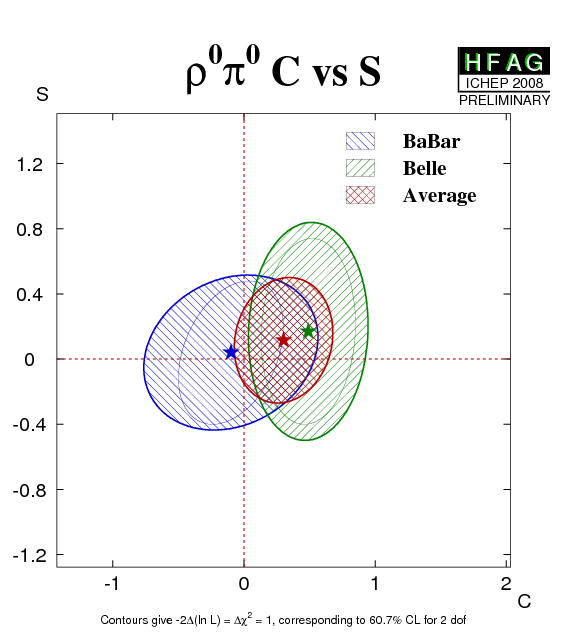
| Experiment | C (ρ0π0) | S (ρ0π0) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=375M |
−0.10 ± 0.40 ± 0.53 | 0.04 ± 0.44 ± 0.18 | 0.35 | PRD 76 (2007) 012004 |
|
Belle
N(BB)=449M |
0.49 ± 0.36 ± 0.28 | 0.17 ± 0.57 ± 0.35 | 0.08 | PRL 98 (2007) 221602 |
| Average | 0.30 ± 0.38 | 0.12 ± 0.38 | 0.12 |
HFAG correlated average
χ2 = 0.5/2 dof (CL=0.76 ⇒ 0.3σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
Interpretations:
The information given above can be used to extract α ≡ φ2.
From the measured form factor bilinears,
BaBar
extract a confidence level interpretation for α,
and constrain α = (87+45−13)° at 68% confidence level.
Belle
has performed a similar analysis.
In addition, Belle
has also included information from the SU(2) partners of B → ρπ,
which can be used to constrain α ≡ φ2 via an
isospin pentagon
relation.
The isospin analysis uses as input the branching fractions and
CP-violating charge asymmetries
of all five ρπ decay modes
(ρ+π−,ρ−π+,
ρ0π0, ρ+π0,
ρ0π+).
The relevant information is taken from
HFAG - Rare Decays and is tabulated below.
[Branching fractions are given in units of 10−6.]
Those values that are not included in the table below
can be obtained from the bilinear form-factors,
eg. a measurement
BR(B0 → ρ0π0)/
BR(B0 → ρ+−π−+) = 0.133 ± 0.022 ± 0.023,
is extracted.
With all information in the ρπ channels put together,
Belle
obtain the tighter constraint
68° < φ2 < 95° at 68% confidence level,
for the solution consistent with the Standard Model.
| BR(B0 → ρ+−π−+) = 23.0 ± 2.3 | - |
| BR(B+ → ρ+π0) = 10.9 +1.4−1.5 | ACP(B+ → ρ+π0) = 0.02 ± 0.11 |
| BR(B+ → π+ρ0) = 8.3 +1.2−1.3 | ACP(B+ → π+ρ0) = 0.18 +0.09−0.17 |
NB. It is implied in the above constraints on α ≡ φ2 that a mirror solution at α → α + π ≡ φ2 → φ2 + π also exists.
For more details on the world average for α ≡ φ2, calculated with different statistical treatments, refer to the CKMfitter and UTfit pages.
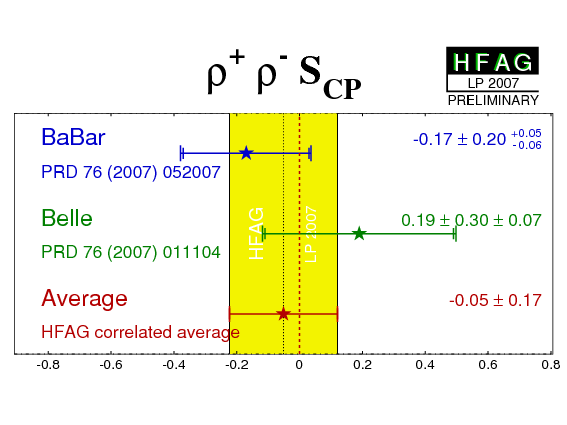
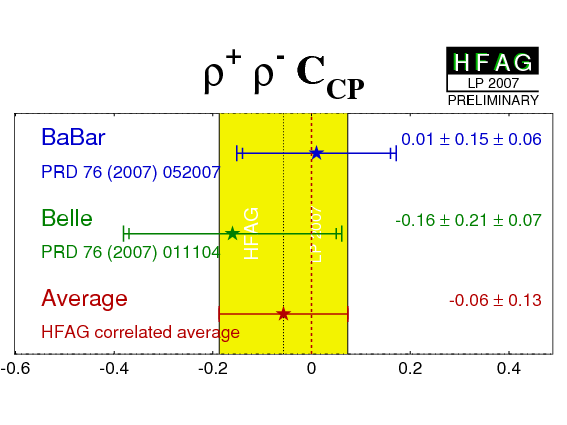
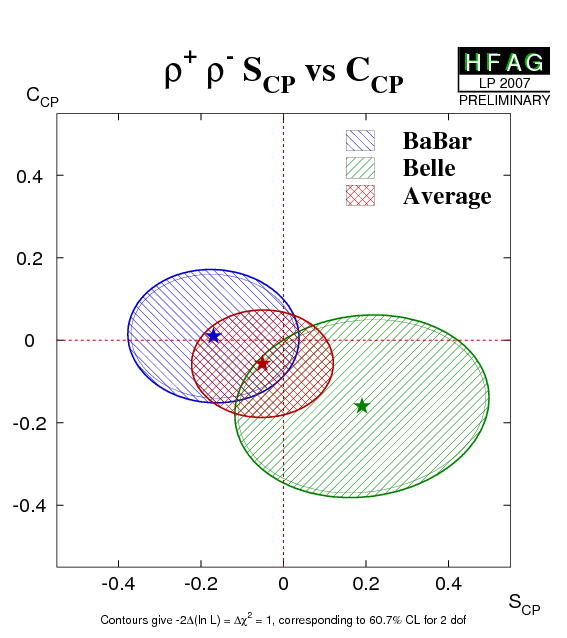
The vector particles in the pseudoscalar to vector-vector decay Bd → ρ+ρ− can have longitudinal and transverse relative polarization with different CP properties. The decay is found to be dominated by the longitudinally polarized component:
At present we do not apply a rescaling of the results to a
common, updated set of input parameters.
The CP parameters measured are those for the longitudinally polarized component
(ie. Sρρ,long, Cρρ,long).
| Experiment | SCP (ρ+ρ−) | CCP (ρ+ρ−) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=387M |
−0.17 ± 0.20 +0.05 −0.06 | 0.01 ± 0.15 ± 0.06 | −0.04 (stat) | PRD 76 (2007) 052007 |
|
Belle
N(BB)=535M |
0.19 ± 0.30 ± 0.07 | −0.16 ± 0.21 ± 0.07 | 0.10 (stat) | PRD 76 (2007) 011104 |
| Average | −0.05 ± 0.17 | −0.06 ± 0.13 | 0.01 |
HFAG correlated average
χ2 = 1.4/2 dof (CL=0.50 ⇒ 0.7σ) |
|
|
eps.gz png |
eps.gz png |
eps.gz png |
|
Since the decay Bd → ρ0ρ0 results in an all charged particle final state, its time-dependent CP violation parameters can be determined experimentally, if difficulties related to the small branching fraction and large backgrounds can be overcome. BaBar have performed the first such analysis. They measure the longitudinally polarized component to be
At present we do not apply a rescaling of the results to a
common, updated set of input parameters.
The CP parameters measured are those for the longitudinally polarized component
(ie. Sρρ,long, Cρρ,long).
| Experiment | SCP (ρ0ρ0) | CCP (ρ0ρ0) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=465M |
0.3 ± 0.7 ± 0.2 | 0.2 ± 0.8 ± 0.3 | 0.0 (stat) | PRD 78 (2008) 071104(R) |
Interpretations:
The
Gronau-London
isospin analysis allows a constraint on α ≡ φ2
to be extracted from the ρρ system even in the presence of non-negligible
penguin contributions.
The analysis involves the SU(2) partners of
the Bd→ ρ+ρ− decay.
The relevant branching ratios (given in units of 10−6)
and CP-violating charge asymmetries are taken from
HFAG - Rare Decays.
| BR(B0 → ρ+ρ−) = 24.2 +3.1−3.2 | — |
| BR(B+ → ρ+ρ0) = 24.0 +1.9−2.0 | ACP(B+ → ρ+ρ0) = −0.051 ± 0.054 |
| BR(B0 → ρ0ρ0) = 0.73 +0.27−0.28 | — |
Belle
obtain φ2 = (88 ± 17)° or
59° < φ2 < 117° at 90% confidence level.
Including an upper limit for the
B0 → ρ0 ρ0 decay,
and world average values for the other parameters, this becomes
φ2 = (91.7 ± 14.9)°.
BaBar
find α ∈ [73, 117]° at 68% confidence level.
In the BaBar analysis of
Bd → ρ0ρ0,
a constraint of |α - αeff| < 15.7° (17.6°)
is obtained at the 68% (90%) CL.
The solution at α - αeff = +11.3° is preferred.
In the BaBar analysis of
B+ → ρ+ρ0,
a constraint of α = (92.4 +6.0−6.5)°
is obtained.
NB. It is implied in the above constraints on α ≡ φ2 that a mirror solution at α → α + π ≡ φ2 → φ2 + π also exists.
For more details on the world average for α ≡ φ2, calculated with different statistical treatments, refer to the CKMfitter and UTfit pages.
The BaBar collaboration have performed a Q2B analysis of the Bd → a1+−π−+ decay, reconstructed in the final state π+π−π+π−.
| Experiment | ACP (a1+−π−+) | C (a1+−π−+) | S (a1+−π−+) | ΔC (a1+−π−+) | ΔS (a1+−π−+) | Correlations | Reference |
|---|---|---|---|---|---|---|---|
|
BaBar
N(BB)=384M |
−0.07 ± 0.07 ± 0.02 | −0.10 ± 0.15 ± 0.09 | 0.37 ± 0.21 ± 0.07 | 0.26 ± 0.15 ± 0.07 | −0.14 ± 0.21 ± 0.06 | (stat) | PRL 98 (2007) 181803 |
|
Belle
N(BB)=772M |
−0.06 ± 0.05 ± 0.07 | −0.01 ± 0.11 ± 0.09 | −0.51 ± 0.14 ± 0.08 | 0.54 ± 0.11 ± 0.07 | −0.09 ± 0.14 ± 0.06 | (stat) | PRD 86 (2012) 092012 |
| Average | −0.06 ± 0.06 | −0.05 ± 0.11 | −0.20 ± 0.13 | 0.43 ± 0.10 | −0.10 ± 0.12 | (stat) |
HFAG correlated average
χ2 = 12/5 dof (CL=0.03 ⇒ 2.1σ) |
|
|
eps.gz png | eps.gz png | eps.gz png | eps.gz png | eps.gz png | . | |
| Experiment | A−+ (a1+−π−+) | A+− (a1+−π−+) | Correlation | Reference |
|---|---|---|---|---|
|
BaBar
N(BB)=384M |
0.07 ± 0.21 ± 0.15 | 0.15 ± 0.15 ± 0.07 | 0.63 (stat) | PRL 98 (2007) 181803 |
|
Belle
N(BB)=772M |
−0.04 ± 0.26 ± 0.19 | 0.07 ± 0.08 ± 0.10 | 0.61 (stat) | PRD 86 (2012) 092012 |
| Average | 0.02 ± 0.20 | 0.10 ± 0.10 | 0.38 |
HFAG correlated average
χ2 = 0.2/2 dof (CL=0.92 ⇒ 0.1σ) |
|
|
eps.gz png | eps.gz png | eps.gz png | |
Interpretations:
The parameter αeff ≡ φ2,eff,
which reduces to α ≡ φ2 in the limit of
no penguin contributions, can be extracted from the above results.
BaBar obtain αeff = (78.6 ± 7.3)°. Belle obtain φ2,eff = (107.3 ± 6.6 (stat) ± 4.8 (syst))°.
NB. There is a four-fold ambiguity in the above results.
For more details on the world average for α ≡ φ2, calculated with different statistical treatments, refer to the CKMfitter and UTfit pages.
Neutral B meson decays such as Bd → D+−π−+, Bd → D*+−π−+ and Bd → D+−ρ−+ provide sensitivity to γ ≡ φ3 because of the interference between the Cabibbo-favoured amplitude (e.g. B0 → D−π+) with the doubly Cabibbo-suppressed amplitude (e.g. B0 → D+π−). The relative weak phase between these two amplitudes is −γ ≡ −φ3 and, when combined with the BdBd-bar mixing phase, the total phase difference is −(2β+γ) ≡ −(2φ1+φ3).
The size of the CP violating effect in each mode depends on the ratio of magnitudes of the suppressed and favoured amplitudes, e.g., rDπ = |A(B0 → D+π−)/A(B0 → D−π+)|. Each of the ratios rDπ, rD*π and rDρ is expected to be about 0.02, and can be obtained experimentally from the corresponding suppressed charged B decays, (e.g., B+ → D+π0) using isospin, or from self-tagging decays with strangeness (e.g., B0 → Ds+π−), using SU(3). In the latter case, the theoretical uncertainties are hard to quantify. The smallness of the r values makes direct extractions from, e.g., the D+−π−+ system very difficult.
Both BABAR and Belle exploit partial reconstructions of D*+−π−+ to increase the available statistics. Both experiments also reconstruct D+−π−+ and D*+−π−+ fully, and BABAR includes the mode D+−ρ−+. Additional states with similar quark content are also possible, but for vector-vector final states an angular analysis is required, while states containing higher resonances may suffer from uncertainties due to nonresonant or other contributions.
BABAR and Belle use different observables:
Here we convert the Belle results to express them in terms of a and c. Explicitly, the conversion reads:
| Belle D*π (partial reconstruction): | aπ* = − (S+ + S−)/2 |
| cπ* = − (S+ − S−)/2 | |
| Belle D*π (full reconstruction): | aπ* = + ( 2 RD*π sin( 2φ1+φ3 + δD*π ) + 2 RD*π sin( 2φ1+φ3 − δD*π ) )/2 |
| cπ* = + ( 2 RD*π sin( 2φ1+φ3 + δD*π ) − 2 RD*π sin( 2φ1+φ3 − δD*π ) )/2 | |
| Belle Dπ (full reconstruction): | aπ = − ( 2 RDπ sin( 2φ1+φ3 + δDπ ) + 2 RDπ sin( 2φ1+φ3 − δDπ ) )/2 |
| cπ = − ( 2 RDπ sin( 2φ1+φ3 + δDπ ) − 2 RDπ sin( 2φ1+φ3 − δDπ ) )/2 |
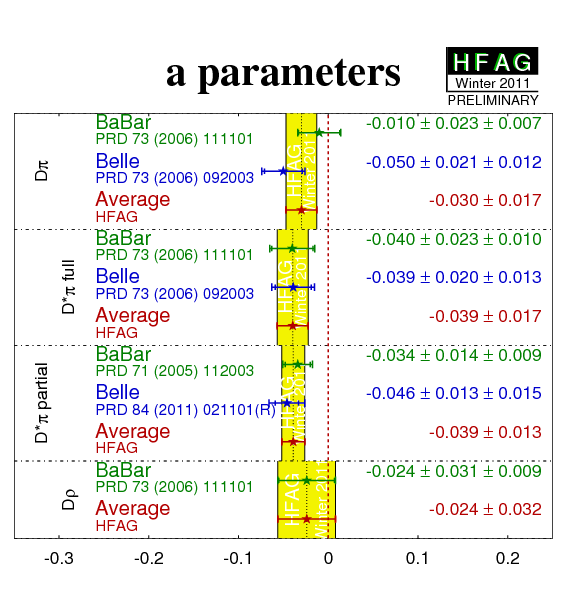
At present we do not rescale the results to a common set of input parameters. Also, common systematic errors are not considered.
| Observable | BABAR | Belle | Average | Reference | ||
|---|---|---|---|---|---|---|
|
partially reconstructed N(BB)=232m |
fully reconstructed N(BB)=232m |
partially reconstructed N(BB)=657m |
fully reconstructed N(BB)=386m |
|||
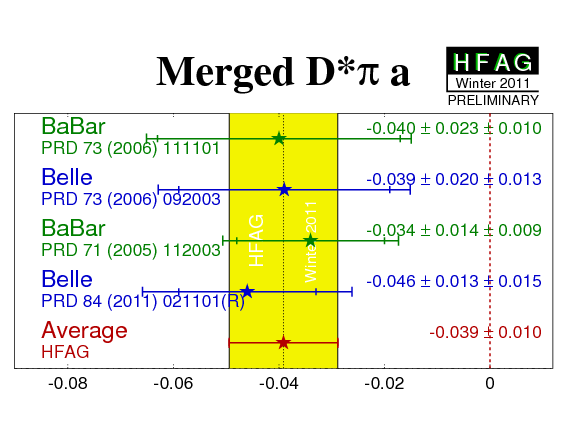
| aD*π | −0.034 ± 0.014 ± 0.009 | −0.040 ± 0.023 ± 0.010 | −0.046 ± 0.013 ± 0.015 | −0.039 ± 0.020 ± 0.013 | −0.039 ± 0.010
CL=0.97 (0.03σ) |
BaBar: PRD 71 (2005) 112003 (partially reco.) BaBar: PRD 73 (2006) 111101 (fully reco.) Belle: PRD 84 (2011) 021101(R) (partially reco.) Belle: PRD 73 (2006) 092003 (fully reco.) |
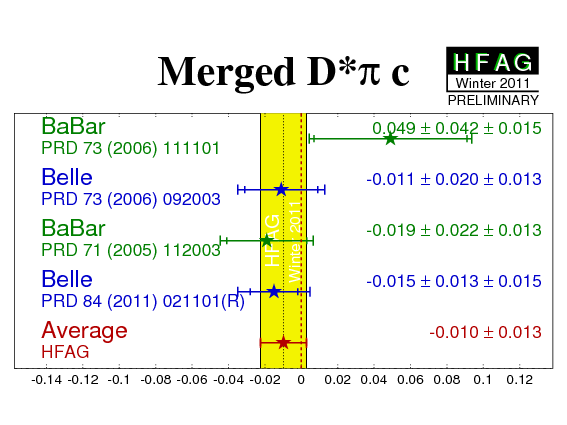
| cD*π | −0.019 ± 0.022 ± 0.013
(lepton tags only) |
0.049 ± 0.042 ± 0.015
(lepton tags only) |
−0.015 ± 0.013 ± 0.015 | −0.011 ± 0.020 ± 0.013 | −0.010 ± 0.013 CL=0.59 (0.6σ) |
|
| aDπ | - | −0.010 ± 0.023 ± 0.007 | - | −0.050 ± 0.021 ± 0.012 | −0.030 ± 0.017
CL=0.24 (1.2σ) |
|
| cDπ | - | −0.033 ± 0.042 ± 0.012
(lepton tags only) |
- | −0.019 ± 0.021 ± 0.012 | −0.022 ± 0.021
CL=0.78 (0.3σ) |
|
| aDρ | - | −0.024 ± 0.031 ± 0.009 | - | - | −0.024 ± 0.033 | |
| cDρ | - | −0.098 ± 0.055 ± 0.018
(lepton tags only) |
- | - | −0.098 ± 0.058 | |
| Compilation of the above results. |
eps.gz png |

eps.gz png |
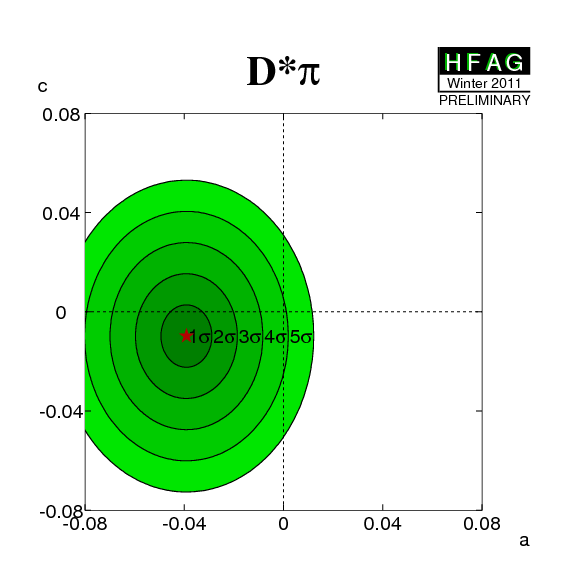
| Averages of the D*π results. |
eps.gz png |
eps.gz png |
Digression:
|
Constraining 2β+γ ≡
2φ1+φ3:
The constraints can be tightened if one is willing to use theoretical input on the values of R and/or δ. One popular choice is the use of SU(3) symmetry to obtain R by relating the suppressed decay mode to B decays involving Ds mesons. For more information, visit the CKMfitter and UTfit sites. |
eps.gz png |
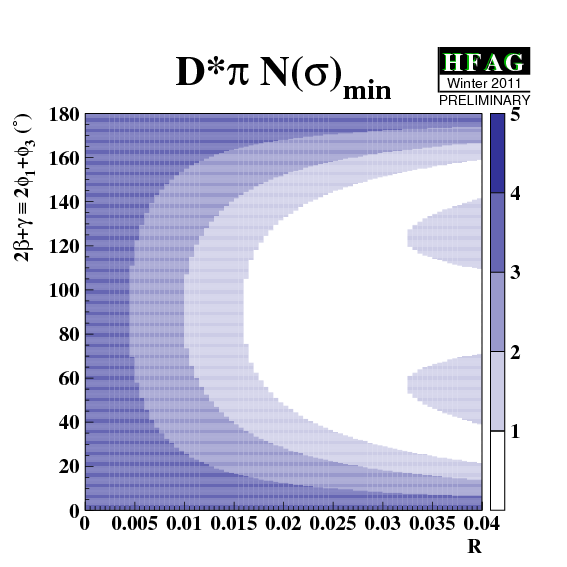
eps.gz png CL: eps.gz png |
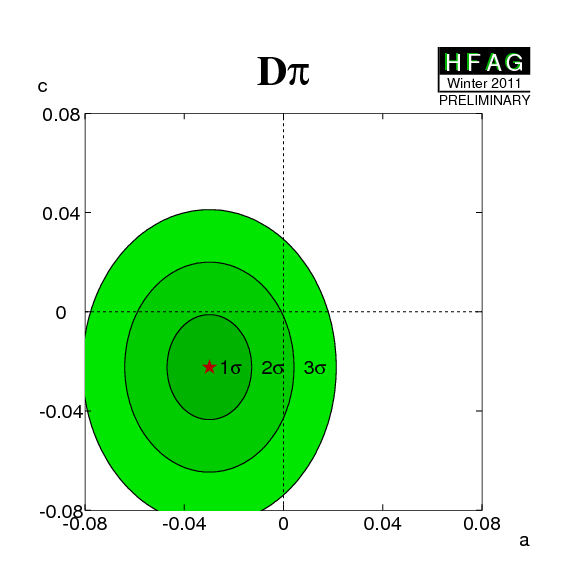
eps.gz png |
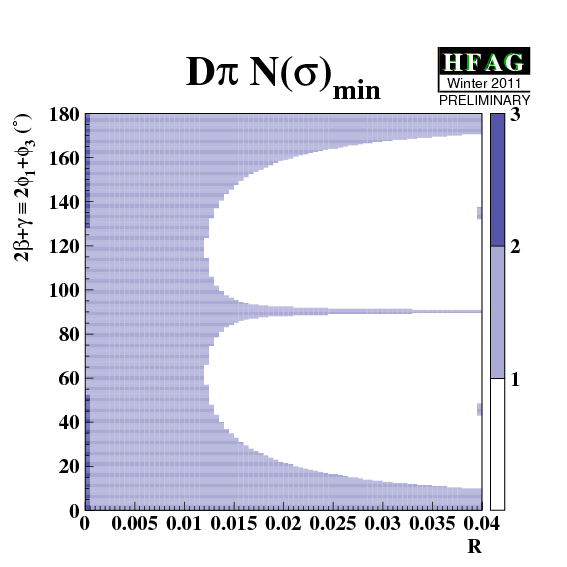
eps.gz png CL: eps.gz png |
Time-dependent analyses of transitions such as Bd → D+−KSπ−+ can be used to probe sin(2β+γ) ≡ sin(2φ1+φ3) in a similar way to that discussed above. Since the final state contains three particles, a Dalitz plot analysis is necessary to maximise the sensitivity. BaBar have carried out such an analysis. They obtain 2β+γ = (83 ± 53 ± 20)° (with an ambiguity 2β+γ → 2β+γ+π) assuming the ratio of the b → u and b → c amplitude to be constant across the Dalitz plot at 0.3.
LHCb have measured the time-dependent CP violation parameters in B0s → D−+sK+− decays.
| Experiment | C | S | Sbar | D | Dbar | Reference |
|---|---|---|---|---|---|---|
|
LHCb
∫Ldt=1 fb−1 |
1.01 ± 0.50 ± 0.23 | −1.25 ± 0.56 ± 0.24 | 0.08 ± 0.68 ± 0.28 | −1.33 ± 0.60 ± 0.26 | −0.81 ± 0.56 ± 0.26 | LHCb-CONF-2012-029 |
A theoretically clean measurement of the angle γ ≡ φ3 can be obtained from the rate and asymmetry measurements of B− → D(*)CPK(*)− decays, where the D(*) meson decays to CP even (D(*)CP+) and CP odd (D(*)CP−) eigenstates. The method benefits from the interference between the dominant b→cu-bar s transitions with the corresponding doubly CKM-suppressed b→uc-bar s transition. It was proposed by Gronau, Wyler and Gronau, London (GLW).
BABAR, Belle, CDF and LHCb use consistent definitions for ACP+− and RCP+−, where
| ACP+− = [Γ(B− → D(*)CP+−K(*)−) − Γ(B+ → D(*)CP+−K(*)+)] / Sum , |
| RCP+− = 2 [Γ(B− → D(*)CP+−K(*)−) + Γ(B+ → D(*)CP+−K(*)+)] / [Γ(B− → D(*)0 K(*)−) + Γ(B+ → D(*)0-bar K(*)+)]. |
Experimentally, it is convenient to measure RCP+− using double ratios, in which similar ratios for B− → D(*) π(*)− decays are used for normalization.
These observables have been measured so far for three D(*)K(*)− modes.
At present we do not rescale the results to a common set of input parameters. Also, common systematic errors are not considered.
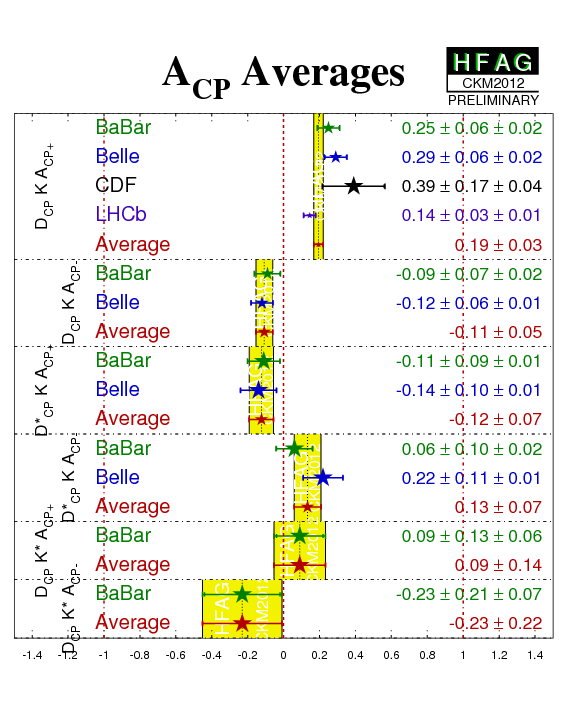
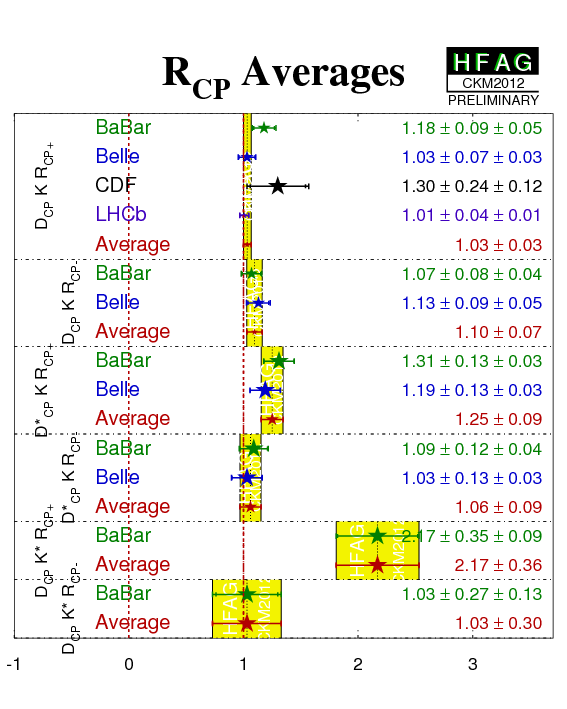
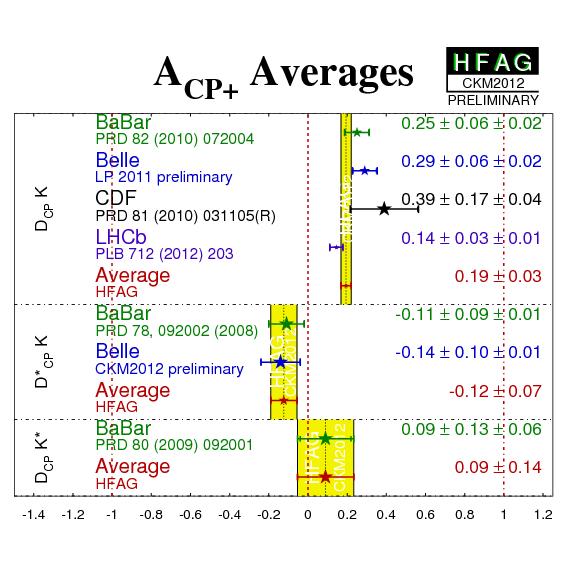
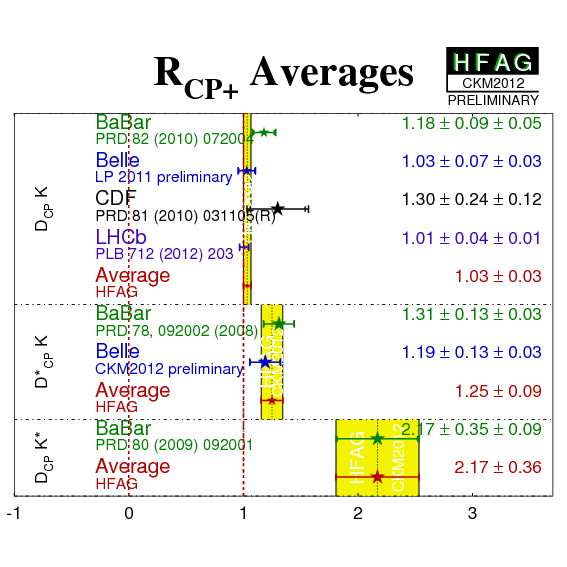
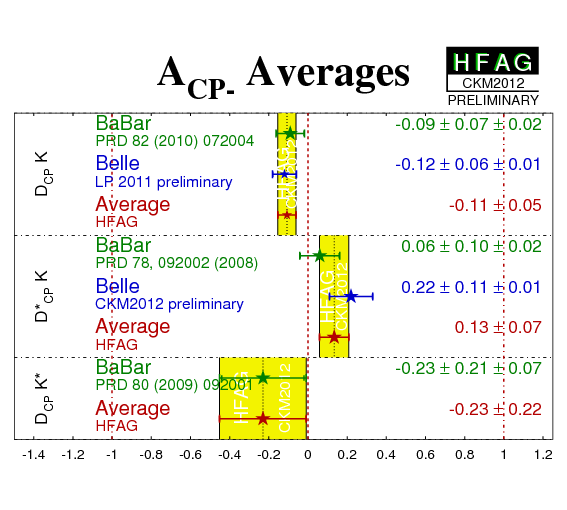
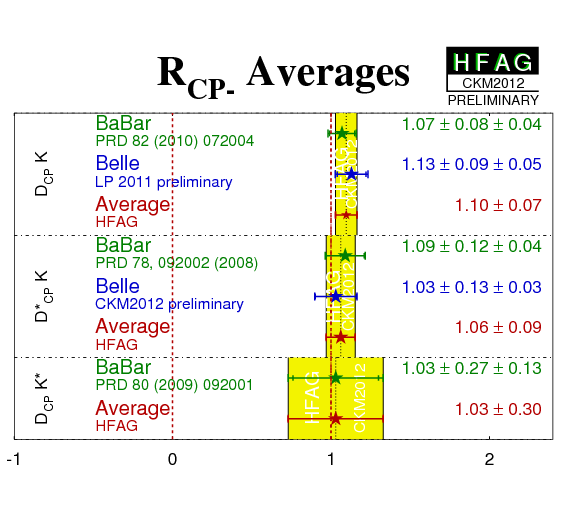
| Mode | Experiment | ACP+ | ACP− | RCP+ | RCP− | Reference |
|---|---|---|---|---|---|---|
| DCPK− |
BaBar
N(BB)=467M |
0.25 ± 0.06 ± 0.02 | −0.09 ± 0.07 ± 0.02 | 1.18 ± 0.09 ± 0.05 | 1.07 ± 0.08 ± 0.04 | PRD 82 (2010) 072004 |
|
Belle
N(BB)=772M |
0.29 ± 0.06 ± 0.02 | −0.12 ± 0.06 ± 0.01 | 1.03 ± 0.07 ± 0.03 | 1.13 ± 0.09 ± 0.05 | LP 2011 preliminary | |
|
CDF
∫Ldt=1 fb−1 |
0.39 ± 0.17 ± 0.04 | - | 1.30 ± 0.24 ± 0.12 | - | PRD 81 (2010) 031105(R) | |
|
LHCb
∫Ldt=1 fb−1 |
0.14 ± 0.03 ± 0.01 | - | 1.01 ± 0.04 ± 0.01 | - | PLB 712 (2012) 203 | |
| Average |
0.19 ± 0.03
χ2 = 6.5/3 dof (CL=0.09 ⇒ 1.7σ) |
−0.11 ± 0.05
χ2 = 0.10 (CL=0.75 ⇒ 0.3σ) |
1.03 ± 0.03
χ2 = 3.5/3 dof (CL=0.33 ⇒ 1.0σ) |
1.10 ± 0.07
χ2 = 0.2 (CL=0.66 ⇒ 0.4σ) |
HFAG | |
|
|
eps.gz png | eps.gz png | eps.gz png | eps.gz png | . | |
| Click here for averages excluding KSφ from the CP-odd channels. | ||||||
| D*CPK− |
BaBar
N(BB)=383M |
−0.11 ± 0.09 ± 0.01 | 0.06 ± 0.10 ± 0.02 | 1.31 ± 0.13 ± 0.03 | 1.09 ± 0.12 ± 0.04 | PRD 78, 092002 (2008) |
|
Belle
N(BB)=772M |
−0.14 ± 0.10 ± 0.01 | 0.22 ± 0.11 ± 0.01 | 1.19 ± 0.13 ± 0.03 | 1.03 ± 0.13 ± 0.03 | CKM2012 preliminary | |
| Average |
−0.12 ± 0.07
χ2 = 0.05 (CL=0.82 ⇒ 0.2σ) |
0.13 ± 0.07
χ2 = 1.1 (CL=0.29 ⇒ 1.1σ) |
1.25 ± 0.09
χ2 = 0.40 (CL=0.52 ⇒ 0.6σ) |
1.06 ± 0.09
χ2 = 0.11 (CL=0.74 ⇒ 0.3σ) |
HFAG | |
|
|
eps.gz png | eps.gz png | eps.gz png | eps.gz png | . | |
| DCPK*− |
BaBar
N(BB)=379M |
0.09 ± 0.13 ± 0.06 | −0.23 ± 0.21 ± 0.07 | 2.17 ± 0.35 ± 0.09 | 1.03 ± 0.27 ± 0.13 | PRD 80 (2009) 092001 |
| Belle | NO RESULTS AVAILABLE (*) | - | ||||
| Average | 0.09 ± 0.14 | −0.23 ± 0.22 | 2.17 ± 0.36 | 1.03 ± 0.30 | HFAG | |
| Compilation of the above results. |
eps.gz png |
eps.gz png |
| CP+ only |
eps.gz png |
eps.gz png |
| CP- only |
eps.gz png |
eps.gz png |
Digression:
Constraining γ ≡ φ3:
The rate ratios and asymmetries of the GLW method can be
expressed in terms of amplitude ratios and strong phase differences,
as well as the weak phase difference γ ≡ φ3.
For the GLW observables, one has:
where rB = |A(b→u)/A(b→c)| and δB = arg[A(b→u)/A(b→c)]. Only the weak phase difference γ ≡ φ3 is universal, while the other parameters depend on the decay process. In addition, the Cartesian coordinates x± (discussed below in the context of analysis of B→DK with multibody D decay) can be extracted from the observables measured in GLW analysis. The relations are
There is no direct sensitivity to y+−, but indirect bounds can be obtained using
|
A modification of the GLW idea has been suggested by Atwood, Dunietz and Soni, where B− → DK− with D → K+π− (or similar) and the charge conjugate decays are used. Here, the favoured (b→c) B decay followed by the doubly CKM-suppressed D decay interferes with the suppressed (b→u) B decay followed by the CKM-favored D decay. The relative similarity of the combined decay amplitudes enhances the possible CP asymmetry. The experiments use consistent definitions for AADS and RADS, where (for example for the B− → DK−, D → K+π− mode)
| AADS = [Γ(B− → [K+π−]DK−) − Γ(B+ → [K−π+]DK+)] / [Γ(B− → [K+π−]DK−) + Γ(B+ → [K−π+]DK+)] , |
| RADS = [Γ(B− → [K+π−]DK−) + Γ(B+ → [K−π+]DK+)] / [Γ(B− → [K−π+]DK−) + Γ(B+ → [K+π−]DK+)] . |
Digression:
|
Recently it has been noted that the observables (R+, R−) may be more suitable for use than (RADS, AADS) since the former are better behaved (they are statistically independent observables, while the uncertainty on AADS depends on the central value of RADS). The definitions are |
|
R+ = Γ(B+ → [K−π+]DK+) / Γ(B+ → [K+π−]DK+) R− = Γ(B− → [K+π−]DK−) / Γ(B− → [K−π+]DK−) |
|
They are related to (RADS, AADS) by |
|
RADS = (R+ + R−)/2 AADS = (R− − R+) / (R− + R+) |
|
We may switch to using this set of variables at a later time, but presently the majority of experimental results are presented in the (RADS, AADS) format. |
(Some of) these observables have been measured so far for the D(*)K(*)− modes. BaBar, Belle, CDF and LHCb have presented results for B− → DK− while BaBar and Belle have also presented results using B− → D*K−, with both D* → Dπ0 and D* → Dγ. BaBar have also presented results on B− → DK*−. For all the above the D → K+π− mode is used. In addition, BaBar have presented results using B− → DK− with D → K+π−π0.
At present we do not rescale the results to a common set of input parameters. Also, common systematic errors are not considered.
| Mode | Experiment | AADS | RADS | Reference | |
|---|---|---|---|---|---|
|
DK−
D→Kπ |
BaBar
N(BB)=467M |
−0.86 ± 0.47 +0.12 −0.16 | 0.011 ± 0.006 ± 0.002 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
−0.39 +0.26 −0.28 +0.04 −0.03 | 0.0163 +0.0044 −0.0041 +0.0007 −0.0013 | PRL 106 (2011) 231803 | ||
|
CDF
∫Ldt=7 fb−1 |
−0.82 ± 0.44 ± 0.09 | 0.0220 ± 0.0086 ± 0.0026 | PRD 84 (2011) 091504 | ||
|
LHCb
∫Ldt=1 fb−1 |
−0.52 ± 0.15 ± 0.02 | 0.0152 ± 0.0020 ± 0.0004 | PLB 712 (2012) 203 | ||
| Average |
−0.54 ± 0.12
χ2 = 1.1/3 dof (CL=0.77 ⇒ 0.3σ) |
0.0153 ± 0.0017
χ2 = 1.1/3 dof (CL=0.78 ⇒ 0.3σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
D*K−
D* → Dπ0 D→Kπ |
BaBar
N(BB)=467M |
0.77 ± 0.35 ± 0.12 | 0.018 ± 0.009 ± 0.004 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
0.4 +1.1 −0.7 +0.2 −0.1 | 0.010 +0.008 −0.007 +0.001 −0.002 | LP 2011 preliminary | ||
| Average |
0.72 ± 0.34
χ2 = 0.1 (CL=0.71 ⇒ 0.4σ) |
0.013 ± 0.006
χ2 = 0.4 (CL=0.52 ⇒ 0.6σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
D*K−
D* → Dγ D→Kπ |
BaBar
N(BB)=467M |
0.36 ± 0.94 +0.25 −0.41 | 0.013 ± 0.014 ± 0.008 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
−0.51 +0.33 −0.29 ± 0.08 | 0.036 +0.014 −0.012 ± 0.002 | LP 2011 preliminary | ||
| Average |
−0.43 ± 0.31
χ2 = 0.7 (CL=0.42 ⇒ 0.8σ) |
0.027 ± 0.010
χ2 = 1.3 (CL=0.26 ⇒ 1.1σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
DK*−
D→Kπ |
BaBar
N(BB)=379M |
−0.34 ± 0.43 ± 0.16 | 0.066 ± 0.031 ± 0.010 | PRD 80 (2009) 092001 | |
|
DK−
D→Kππ0 |
BaBar
N(BB)=474M |
- | 0.0091 +0.0082 −0.0076 +0.0014 −0.0037 | PRD 84 (2011) 012002 | |
|
DK−
D→K3π |
LHCb
∫Ldt=1 fb−1 |
−0.42 ± 0.22 | 0.0124 ± 0.0027 | LHCb-CONF-2012-030 | |
| Compilation of the above results. |
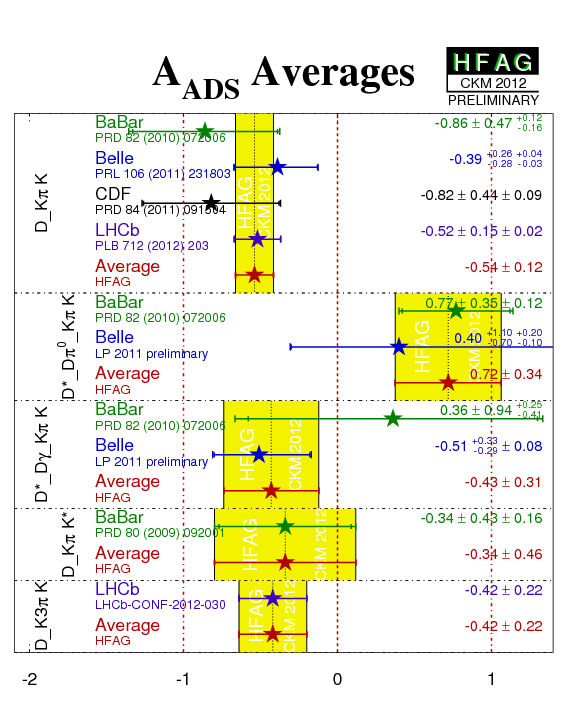
eps.gz png |

eps.gz png |
Digression:
|
Constraining γ ≡ φ3: As for the GLW method, the rate ratios and asymmetries of the ADS method can be expressed in terms of amplitude ratios and strong phase differences, as well as the weak phase difference γ ≡ φ3. For the ADS observables, one has:
where rB = |A(b→u)/A(b→c)| and δB = arg[A(b→u)/A(b→c)] as before. rD and δD are the corresponding amplitude ratio and strong phase difference of the D meson decay amplitudes. The value of rD2 is obtained from the ratio of the suppressed-to-allowed branching fractions, ensuring that mixing effects are corrected for. The value of δD can be determined directly using quantum correlated D mesons produced in ψ(3770) decay, as has been done by CLEO. The most precise values of both quantities come from the averages performed by HFAG to obtain the mixing parameters in the charm system. The strong phase, δB, is different, in general, for decays to D and D* mesons. Bondar and Gershon have pointed out that there is an effective strong phase shift of π between the cases that D* is reconstructed in the Dπ0 and Dγ final states, which in principle allows γ ≡ φ3 to be measured using the ADS technique with B+− → D* K+− alone. The situation for D→Kππ0 is slightly more complicated since the hadronic parameters can vary across the phase space (Dalitz plane). Effective hadronic parameters can be used, and eventually a Dalitz analysis (either binned or unbinned) may be possible to extract the maximum information from the decay. |
As can be seen from the expressions above, the maximum size of the asymmetry, for given values of rB and rD is given by: AADS (max) = 2rBrD / (rB2+rD2). Thus, sizeable asymmetries may be found also for B− → D(*)π− decays, despite the expected smallness (~0.01) of rB for this case, providing sensitivity to γ ≡ φ3. Some of the observables have been measured by BABAR, Belle, CDF and LHCb in the various D(*)π− modes.
| Mode | Experiment | AADS | RADS | Reference | |
|---|---|---|---|---|---|
|
Dπ−
D→Kπ |
BaBar
N(BB)=467M |
0.03 ± 0.17 ± 0.04 | 0.0033 ± 0.0006 ± 0.0004 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
−0.04 ± 0.11 +0.02 −0.01 | 0.00328 +0.00038 −0.00036 +0.00012 −0.00018 | PRL 106 (2011) 231803 | ||
|
CDF
∫Ldt=7 fb−1 |
0.13 ± 0.25 ± 0.02 | 0.0028 ± 0.0007 ± 0.0004 | PRD 84 (2011) 091504 | ||
|
LHCb
∫Ldt=1 fb−1 |
0.143 ± 0.062 ± 0.011 | 0.00410 ± 0.00025 ± 0.00005 | PLB 712 (2012) 203 | ||
| Average |
0.09 ± 0.05
χ2 = 2.2/3 dof (CL=0.53 ⇒ 0.6σ) |
0.00375 ± 0.00020
χ2 = 5.1/3 dof (CL=0.17 ⇒ 1.4σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
D*π−
D* → Dπ0 D→Kπ |
BaBar
N(BB)=467M |
−0.09 ± 0.27 ± 0.05 | 0.0032 ± 0.0009 ± 0.0008 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
−0.07 ± 0.23 ± 0.05 | 0.0040 +0.0010 −0.0009 ± 0.0003 | LP 2011 preliminary | ||
| Average |
−0.08 ± 0.18
χ2 = 0.003 (CL=0.96 ⇒ 0.1σ) |
0.0037 ± 0.0008
χ2 = 0.27 (CL=0.61 ⇒ 0.5σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
D*π−
D* → Dγ D→Kπ |
BaBar
N(BB)=467M |
−0.65 ± 0.55 ± 0.22 | 0.0027 ± 0.0014 ± 0.0022 | PRD 82 (2010) 072006 | |
|
Belle
N(BB)=772M |
−0.10 +0.26 −0.25 ± 0.02 | 0.0041 +0.0011 −0.0010 ± 0.0001 | LP 2011 preliminary | ||
| Average |
−0.19 ± 0.23
χ2 = 0.73 (CL=0.39 ⇒ 0.9σ) |
0.0039 ± 0.0010
χ2 = 0.25 (CL=0.62 ⇒ 0.5σ) |
HFAG | ||
|
|
eps.gz png | eps.gz png | . | ||
|
Dπ−
D → K3π |
LHCb
∫Ldt=1 fb−1 |
0.130 ± 0.097 | 0.00369 ± 0.00036 | LHCb-CONF-2012-030 | |
| Compilation of the above results. |
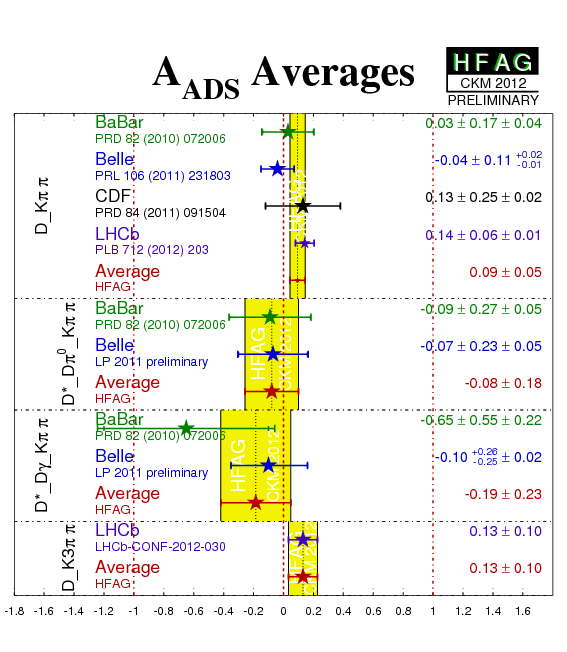
eps.gz png |
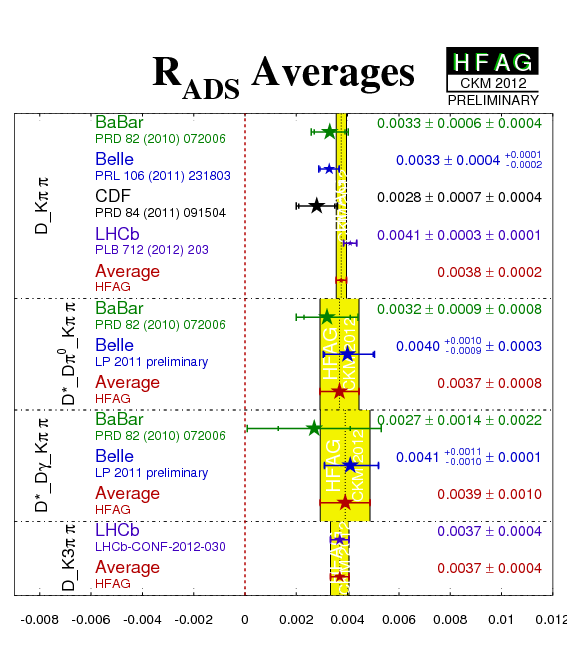
eps.gz png |
Another method to extract γ ≡ φ3 from the interference between B− → D(*)0 K− and B− → D(*)0-bar K− uses multibody D decays. A Dalitz plot analysis allows simultaneous determination of the weak phase difference γ ≡ φ3, the strong phase difference δB and the ratio of amplitudes rB. This idea was proposed by Giri, Grossman, Soffer and Zupan and the Belle Collaboration. The assumption of a D decay model results in an additional model uncertainty. (See below for results of a model-independent approach to the analysis.)
Results are available from both Belle and BaBar using B− → D K−, B− → D*K− and B− → DK*−. Both BaBar and Belle use both D* decays to Dπ0 and Dγ, taking the effective strong phase shift into account. Both experiments use the decay D → KSπ+π−; BaBar also use D → KSK+K− (though not for B− → DK*−).
For the DK*− mode, both collaborations use K*− → KSπ−; in this case some care is needed due to other possible contributions to the B− → DKSπ− final state. Belle assign an additional (model) uncertainty, while BaBar using use an alternative parametrization [replacing rB and δB with κrs and δs, respectively] suggested by Gronau.
If the values of γ ≡ φ3, δB and rB are obtained by directly fitting the data, the extracted value of rB is biased (since it is positive definite by nature). Since the error on γ ≡ φ3 depends on the value of rB some statistical treatment is necessary to correctly estimate the uncertainty. To obviate this effect, both experiments now use a different set of variables in the fits:
| x+ = rB cos( δB+γ ) ≡ rB cos( δB+φ3 ) | y+ = rB sin( δB+γ ) ≡ rB sin( δB+φ3 ) |
| x− = rB cos( δB−γ ) ≡ rB cos( δB−φ3 ) | y− = rB sin( δB−γ ) ≡ rB sin( δB−φ3 ) |
Note that (x+,y+) are determined from B+ decays, while (x−,y−) are determined from B− decays.
These parameters have the advantage of having (approximately) Gaussian distributions, and of having small statistical correlations. Some statistical treatment is necessary to convert these measurements into constraints on the underlying physical parameters γ ≡ φ3, δB and rB [BaBar do not obtain constraints on rB and δB for the B− → DK*− decay due to the reparametrization described above]. Both experiments use frequentist procedures, though there are differences in the details.
The results below have three sets of errors, which are statistical, systematic, and model related uncertainties respectively. For details of correlations in the model uncertainty assigned by Belle, (See Appendix of Ref.) The Belle results also include an additional source of uncertainty due to background from B− → DKSπ− other than B− → DK*−, which we have not included here.
Averages are performed using the following procedure.
| Mode | Experiment | x+ | y+ | x- | y- | Correlation | Reference |
|---|---|---|---|---|---|---|---|
| DK− |
BaBar
N(BB)=468M |
−0.103 ± 0.037 ± 0.006 ± 0.007 | −0.021 ± 0.048 ± 0.004 ± 0.009 | 0.060 ± 0.039 ± 0.007 ± 0.006 | 0.062 ± 0.045 ± 0.004 ± 0.006 | (stat) (syst) (model) | PRL 105 (2010) 121801 |
|
Belle
N(BB)=657M |
−0.107 ± 0.043 ± 0.011 ± 0.055 | −0.067 ± 0.059 ± 0.018 ± 0.063 | 0.105 ± 0.047 ± 0.011 ± 0.064 | 0.177 ± 0.060 ± 0.018 ± 0.054 | (stat) (model) | PRD 81 (2010) 112002 | |
| Average No model error |
−0.104 ± 0.029 | −0.038 ± 0.038 | 0.085 ± 0.030 | 0.105 ± 0.036 | (stat+syst) |
HFAG correlated average
χ2 = 3.6/4 dof (CL=0.47 ⇒ 0.7σ) |
|
|
NB. The contours in these plots do not include model errors. |
eps.gz png |
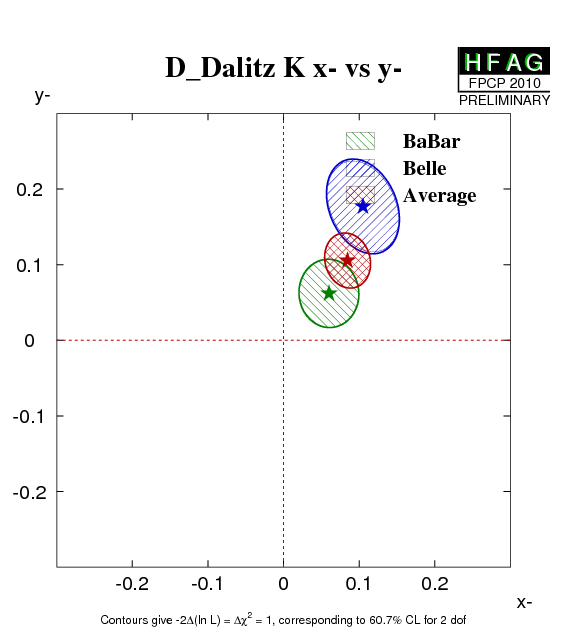
eps.gz png |
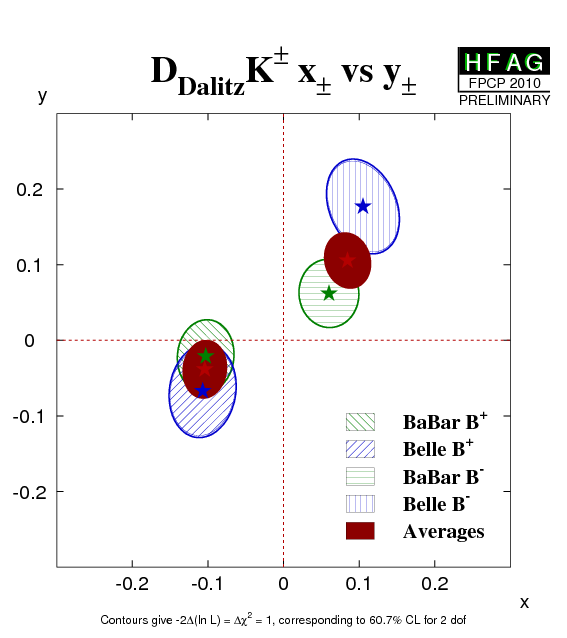
eps.gz png |
||||
| D*K− |
BaBar
N(BB)=468M |
0.147 ± 0.053 ± 0.017 ± 0.003 | −0.032 ± 0.077 ± 0.008 ± 0.006 | −0.104 ± 0.051 ± 0.019 ± 0.002 | −0.052 ± 0.063 ± 0.009 ± 0.007 | (stat) (syst) (model) | PRL 105 (2010) 121801 |
|
Belle
(*)
N(BB)=657M |
0.083 ± 0.092 ± 0.081 | 0.157 ± 0.109 ± 0.063 | −0.036 ± 0.127 ± 0.090 | −0.249 ± 0.118 ± 0.049 | (stat) (model) | PRD 81 (2010) 112002 | |
| Average No model error |
0.130 ± 0.048 | 0.031 ± 0.063 | −0.090 ± 0.050 | −0.099 ± 0.056 | (stat+syst) |
HFAG correlated average
χ2 = 5.0/4 dof (CL=0.29 ⇒ 1.1σ) |
|
|
NB. The contours in these plots do not include model errors. |
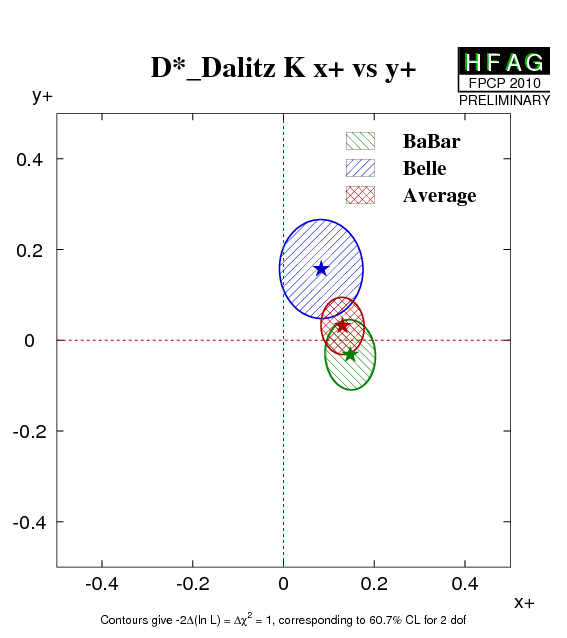
eps.gz png |
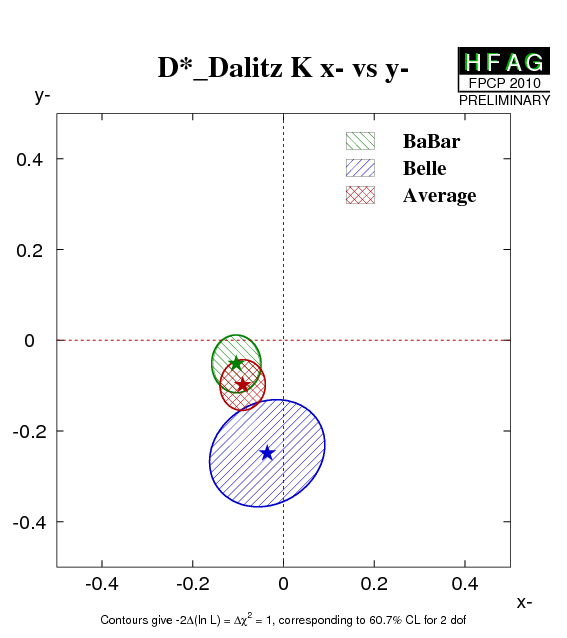
eps.gz png |
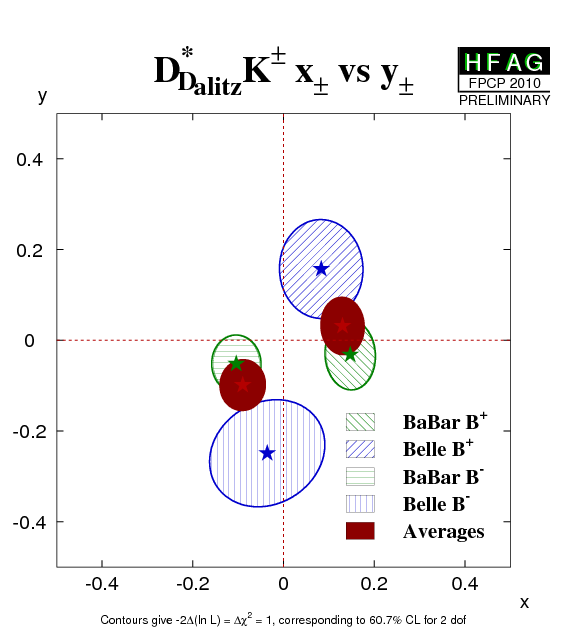
eps.gz png |
||||
| DK*− |
BaBar
N(BB)=468M |
−0.151 ± 0.083 ± 0.029 ± 0.006 | 0.045 ± 0.106 ± 0.036 ± 0.008 | 0.075 ± 0.096 ± 0.029 ± 0.007 | 0.127 ± 0.095 ± 0.027 ± 0.006 | (stat) (syst) (model) | PRL 105 (2010) 121801 |
|
Belle
N(BB)=386M |
−0.105 +0.177 −0.167 ± 0.006 ± 0.088 | −0.004 +0.164 −0.156 ± 0.013 ± 0.095 | −0.784 +0.249 −0.295 ± 0.029 ± 0.097 | −0.281 +0.440 −0.335 ± 0.046 ± 0.086 | (stat) (model) | PRD 73, 112009 (2006) | |
| Average No model error |
−0.152 ± 0.077 | 0.024 ± 0.091 | −0.043 ± 0.094 | 0.091 ± 0.096 | (stat+syst) |
HFAG correlated average
χ2 = 13/4 dof (CL=0.011 ⇒ 2.5σ) |
|
|
NB. The contours in these plots do not include model errors. |
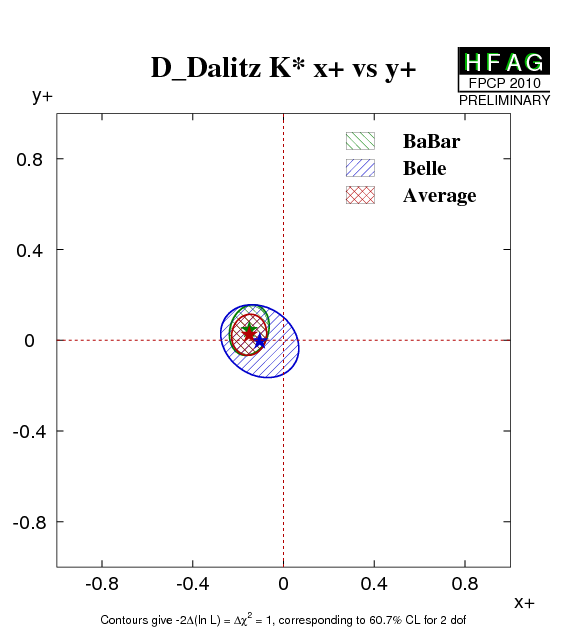
eps.gz png |
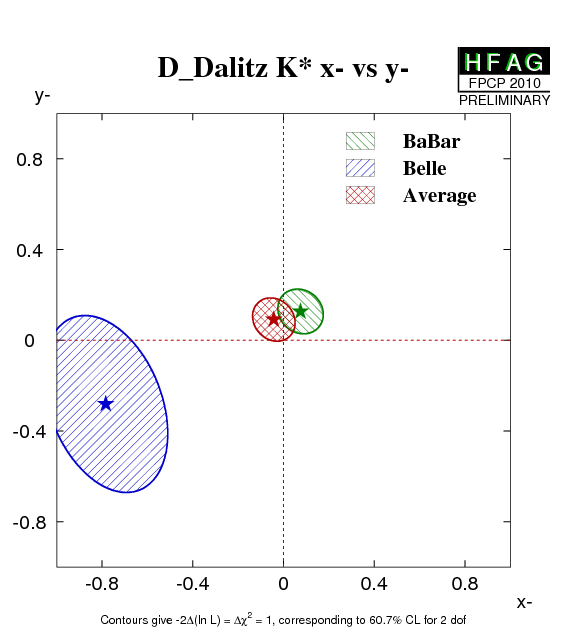
eps.gz png |
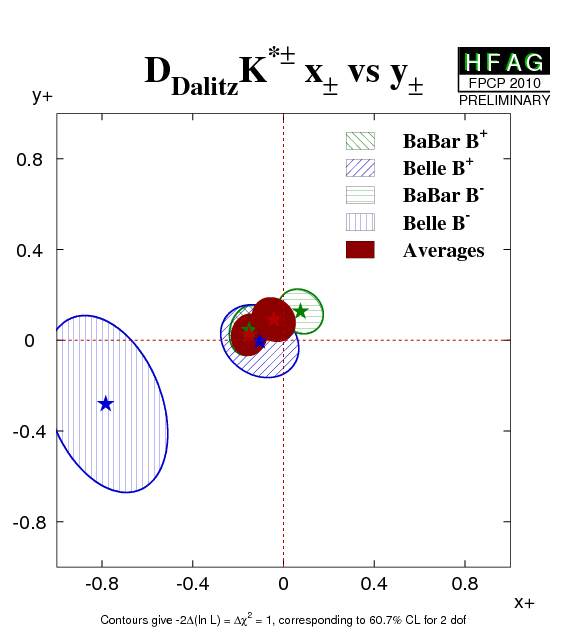
eps.gz png |
||||
|
Compilation of (x±,y±) measurements from B → D(*)K(*) decays with D → KSπ+π− and D → KSK+K−. NB. The uncertainities in these plots do not include model errors. |
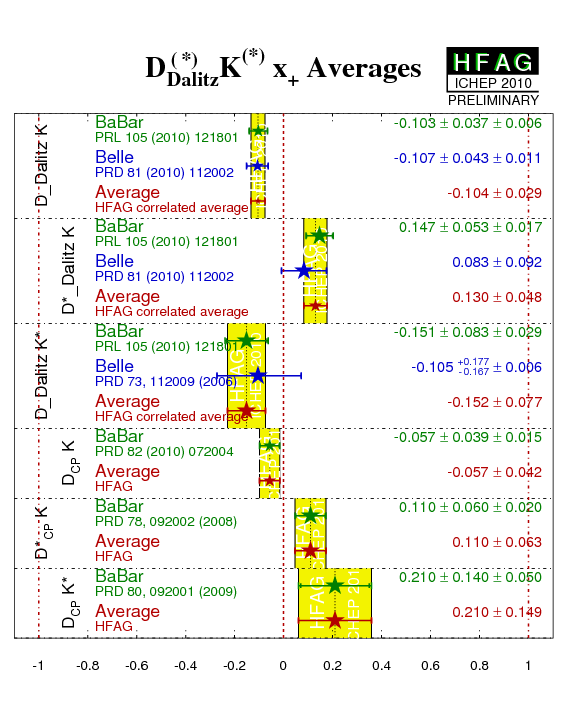
eps.gz png |
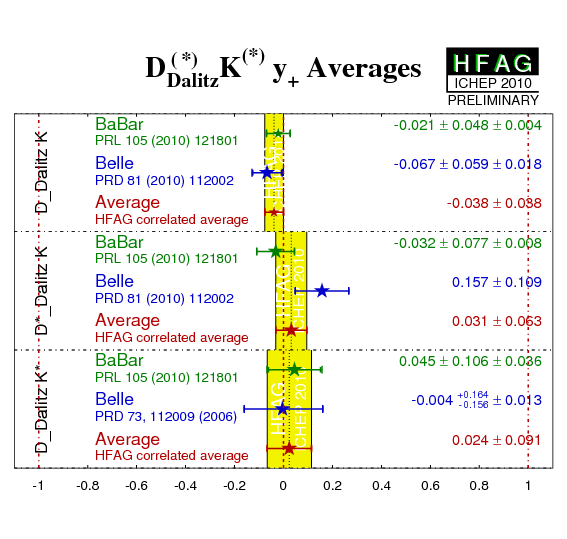
eps.gz png |

eps.gz png |
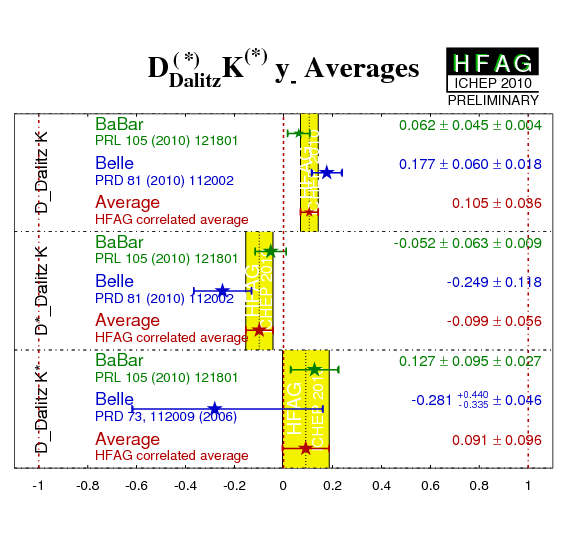
eps.gz png |
|
Compilation of x+ and x− measurements including results from Dalitz and GLW analyses. NB. The uncertainities in these plots do not include model errors. |
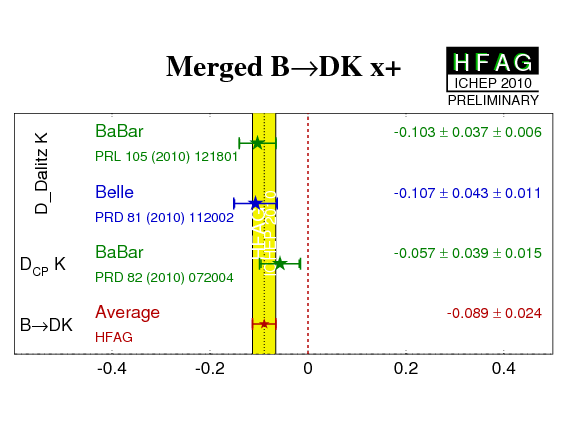
eps.gz png |
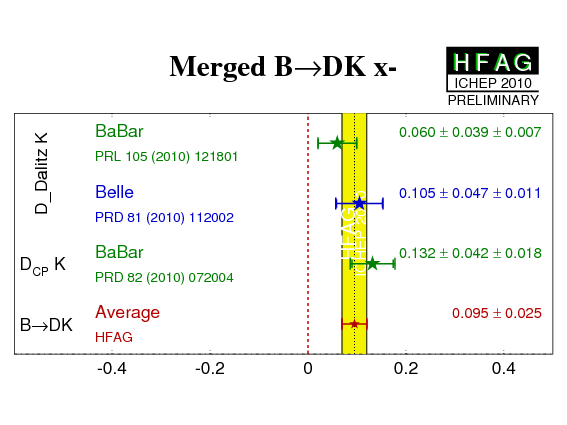
eps.gz png |
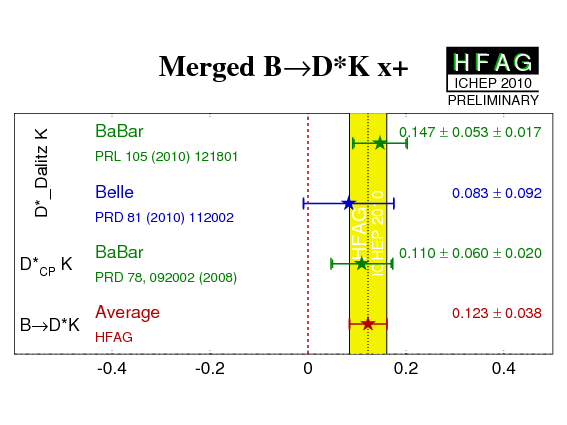
eps.gz png |
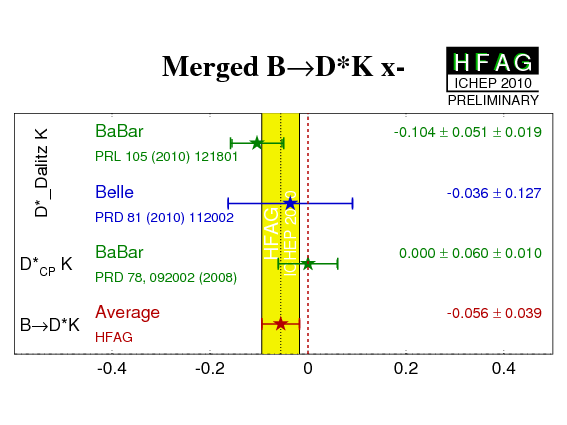
eps.gz png |
| . |
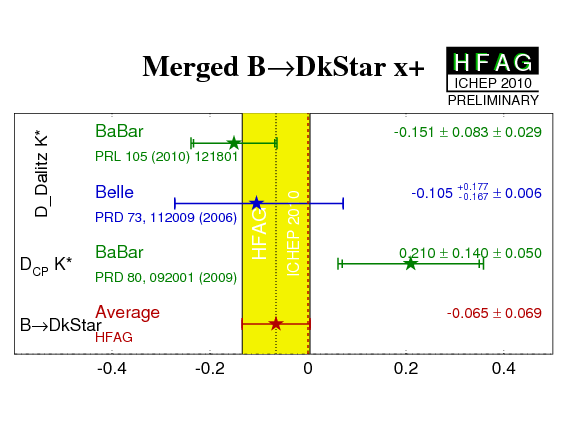
eps.gz png |
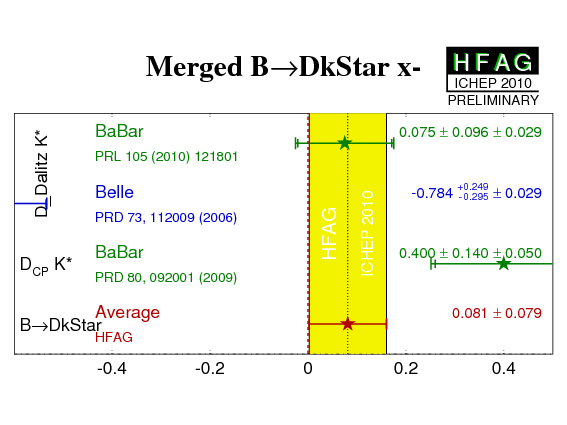
eps.gz png |
. |
Digression:
|
Constraining γ ≡ φ3:
The measurements of x+,− and y+,− in the various D(*)K(*) decay modes can be used to place bounds on γ ≡ φ3. Both experiments have done so using frequentist techniques. |
|||
|
BaBar obtain
γ = (68 +15−14 ± 4 ± 3)° (from DK−, D*K− & DK*−) |
Belle obtain
φ3 = (78 +11−12 ± 4 ± 9)° (from DK− & D*K−) |
||
| The experiments also obtain values for the hadronic parameters | |||
| rB (DK−) = 0.096 ± 0.029 ± 0.005 ± 0.004 | δB (DK−) = (119 +19−20 ± 3 ± 3)° | rB (DK−) = 0.160 +0.040−0.038 ± 0.011+0.05−0.010 | δB (DK−) = (138 +13−16 ± 4 ± 23)° |
| rB (D*K−) = 0.133 +0.042−0.039 ± 0.014 ± 0.003 | δB (D*K−) = (−82 ± 21 ± 5 ± 3)° | rB (D*K−) = 0.196 +0.072−0.069 ± 0.012 +0.062−0.012 | δB (D*K−) = (342 +19−21 ± 3 ± 23)° |
| κrs = 0.149 +0.066−0.062 ± 0.026 ± 0.006 | δs = (111 ± 32 ± 11 ± 3)° | ( rB (DK*−) = 0.56 +0.22−0.16 ± 0.04 ± 0.08 *) | (δ B (DK*−) = (243+20−23 ± 3 ± 50 )° *) |
| Note that the above results suffer an ambiguity: γ → γ + π ≡ φ3 → φ3 + π, δ → δ + π. We quote the result which is consistent with the Standard Model fit. | |||
A model-independent approach to the analysis of B− → D(*) K− with multibody D decays was proposed by Giri, Grossman, Soffer and Zupan, and further developed by Bondar and Poluektov (see also here). The method relies on information on the average strong phase difference between D0 and D0-bar decays in bins of Dalitz plot position that can be obtained from quantum-correlated Ψ(3770) → D0D0-bar events. This information is measured in the form of parameters ci and si that are the amplitude weighted averages of the cosine and sine of the strong phase difference in a Dalitz plot bin labelled by i, respectively. These quantities have been obtained for D → KSπ+π− (and D → KSK+K−) by CLEOc (see also here).
Model-independent determinations of γ ≡ φ3 has been performed by Belle and LHCb using B− → D K− with D → KSπ+π−. The LHCb result also includes D → KSK+K−, but we have not included that in our compilation for now. The variables (x±, y±), defined above are determined from the data. Note (in the Belle case) that due to the strong statistical and systematic correlations with the model-dependent results given above, these results cannot be combined.
The results below have three sets of errors, which are statistical, systematic, and uncertainty coming from the knowledge of ci and si respectively. To perform the average, we remove the last uncertainty, which should be 100% correlated between the measurements. Since the size of the uncertainty from ci and si is found to depend on the size of the B → DK data sample, we assign the smaller of the Belle and LHCb uncertainties to the averaged result. This procedure should be conservative.
| Mode | Experiment | x+ | y+ | x- | y- | Correlation | Reference | ||
|---|---|---|---|---|---|---|---|---|---|
|
DK−
D→KSπ+π− |
Belle
N(BB)=772M |
−0.110 ± 0.043 ± 0.014 ± 0.007 | −0.050 +0.052 −0.055 ± 0.011 ± 0.007 | 0.095 ± 0.045 ± 0.014 ± 0.010 | 0.137 +0.053 −0.057 ± 0.015 ± 0.023 | (stat) | PRD 85 (2012) 112014 | ||
|
LHCb
∫Ldt=1 fb−1 |
−0.086 ± 0.054 ± 0.017 ± 0.016 | −0.003 ± 0.037 ± 0.009 ± 0.027 | 0.016 ± 0.048 ± 0.014 ± 0.008 | 0.014 ± 0.054 ± 0.008 ± 0.024 | (stat) (syst) | PLB 718 (2012) 43 | |||
| Average | −0.101 ± 0.035 ± 0.007 | −0.019 ± 0.031 ± 0.007 | 0.064 ± 0.034 ± 0.010 | 0.078 ± 0.039 ± 0.023 | (stat) |
HFAG correlated average
χ2 = 5.2/4 dof (CL=0.26 ⇒ 1.1σ) |
|||
|
NB. The contours in these plots do not include model errors. |

eps.gz png |
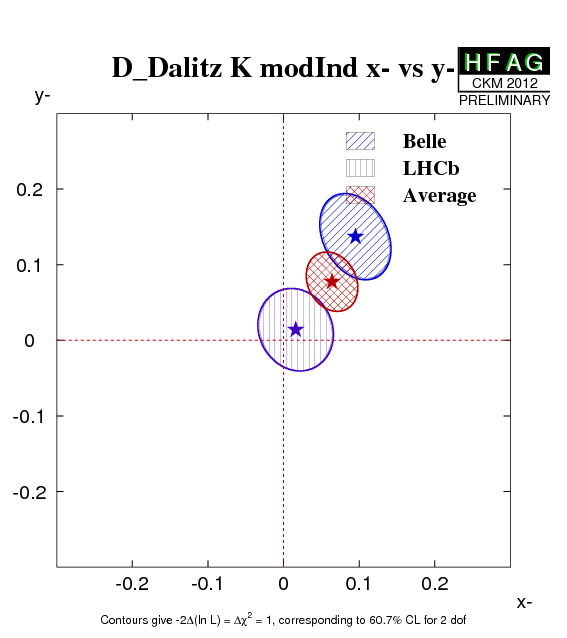
eps.gz png |

eps.gz png |
. | |||||
Digression:
|
Constraining γ ≡ φ3:
As above, the measurements of x+,− and y+,− can be used to place bounds on γ ≡ φ3. Belle and LHCb have done so using a frequentist technique. |
|||
|
Belle obtain
φ3 = (77.3 +15.1−14.9 ± 4.1 ± 4.3)° |
LHCb obtain
γ = (45 +43−38)° |
||
| rB (DK−) = 0.145 ± 0.030 ± 0.010 ± 0.011 | δB (DK−) = (129.9 ± +15.0 ± 3.8 ± 4.7)° | rB (DK−) = 0.07 ± 0.04 | δB (DK−) = (138 +35−46 )° |
| Note that the above results suffer an ambiguity: γ → γ + π ≡ φ3 → φ3 + π, δ → δ + π. We quote the result which is consistent with the Standard Model fit. | |||
BaBar have performed a similar Dalitz plot analysis using the decay D → π+π−π0. In this case the measured yields of B− → DK− and B+ → DK+ events are found to make a significant contribution to the sensitivity to CP violation and this information is included into the fit. Consequently, an alternative set of fit parameters is used in order to avoid significant biasing and nonlinear correlations. The result is parameterized in terms of polar coordinates:
| ρ± ≡ | z± - x0 | | θ± ≡ tan− 1 (Im(z±) / (Re(z±) - x0)) |
where the constant x0 = 0.850 depends on the amplitude structure of the D → π+π−π0 decay, and z± = rB ei( δB ± γ ) ≡ rB ei( δB ± φ3 ). This choice of variables is motivated by the fact that the yields of B± decays are proportional to 1 + ρ±2 - x02. The uncertainty due to the D decay model is included in the systematic error.
| Mode | Experiment | ρ+ | θ+ | ρ− | θ− | Reference |
|---|---|---|---|---|---|---|
|
DK−
D→ π+π−π0 |
BaBar
N(BB)=324M |
0.75 ± 0.11 ± 0.04 | (147 ± 23 ± 1)° | 0.72 ± 0.11 ± 0.04 | (173 ± 42 ± 2)° | PRL 99 (2007) 251801 |
| Average | 0.75 ± 0.12 | (147 ± 23)° | 0.72 ± 0.12 | (173 ± 42)° |
Digression:
|
Constraining γ ≡ φ3:
The measurements of ρ+,− and θ+,− can be used to place bounds on γ ≡ φ3 and the hadronic parameters. BaBar use a frequentist technique to obtain −30° < γ < 76°, 0.06 < rB (DK−) < 0.78 and −27° < δ B (DK−) < 78° at the 68% confidence level. |
LHCb have presented results on B0 → DK*0 with D → K+K−
| Experiment | ACP+ | RCP+ | Reference |
|---|---|---|---|
|
LHCb
∫Ldt=1 fb−1 |
−0.47 +0.24 −0.25 ± 0.02 | 1.42 +0.41 −0.35 ± 0.07 | arXiv:1212.5205 |
BaBar have presented results on B0 → DK*0 with D → K−π+, D → K−π+ π0 and D → K−π+ π+ π−. Belle have presented results with the D → K−π+ mode. The following 95% CL limits are set:
|
BaBar
N(BB)=465M |
RADS(Kπ) < 0.244 | RADS(Kππ0) < 0.181 | RADS(Kπππ) < 0.391 | PRD 80 (2009) 031102 |
|
Belle
N(BB)=772M |
RADS(Kπ) < 0.16 | - | - | PRD 86 (2012) 011101 |
(See above for a definition of the parameters).
| Combining the results and using additional input from CLEOc (here and here), BaBar set a limit on the ratio between the b→u and b→c amplitudes of rs ∈ [0.07,0.41] at 95% CL. |
BaBar have performed a similar Dalitz plot analysis to that described above using neutral B decays. In order to avoid complications due to B0–B0-bar oscillations (see here), the decay to the self-tagging final state DK*0, with K*0 → K+π−, is used. Effects due to the natural width of the K*0 are handled using the parametrization suggested by Gronau.
|
Constraining γ ≡ φ3:
BaBar extract the three-dimensional likelihood for the parameters (γ, δS, rS) and, combining with a separately measured PDF for rS (using a Bayesian technique), obtain bounds on each of the three parameters. |
| γ = (162 ± 56)°, δS = (62 ± 57)° rS < 0.55 at 95% probability |
| Note that there is an ambiguity in the solutions for γ and δS (γ, δS → γ+π, δS+π). |
LHCb have presented results on B+ → DK+ π+π− with D → K+K− and π+π−
| Experiment | ACP+ | RCP+ | Reference |
|---|---|---|---|
|
LHCb
∫Ldt=1 fb−1 |
−0.14 ± 0.10 ± 0.01 | 0.95 ± 0.11 ± 0.02 | LHCb-CONF-2012-021 |
BaBar, Belle and LHCb have all presented constraints on γ ≡ φ3 from combinations of their results on B+ → DK+ and related processes.
|
BaBar obtain γ = (69 +17−16)° rB = 0.092 +0.013−0.012 δB = (105 +16−17)° arXiv:1301.1029 |
Belle obtain φ3 = (68 +15−14)° rB = 0.112 +0.014−0.015 δB = (116 +18−21)° CKM2012 preliminary |
LHCb obtain γ = (71.1 +16.6−15.7)° rB = 0.0919 +0.0083−0.0082 δB = (112.0 +12.6−15.5)° LHCb-CONF-2012-032 |
For attempts to extract γ ≡ φ3 from all available experimental results, visit the CKMfitter and UTfit sites.